Student Essays and Reports

The fullerene family and fullerene-based materials

Fullerenes are a family of a series of hollow molecules that consists of carbon only. Fullerenes are the third well-defined allotrope of carbon, the other two being diamond and graphite. Fullerenes can be divided into three classes on the basis of the shape. Spherical and cylinder-shaped fullerenes are also known as 'buckyballs' and 'nanotubes', respectively (see Figure 1). The third class of fullerenes has a shape of an ellipsoid. [1] In many occasions the buckyballs are called simply as fullerenes.

Fullerenes were officially discovered in 1985 by H.W. Kroto, R.E. Smalley, and R.F. Curl Jr. whose paper reported the discovery of the spherical carbon molecule consisting of 60 atoms, i.e. C₆₀. [2] The new large carbon molecule was named buckminsterfullerene after the American architect R. Buckminster Fuller who designed a geodesic dome that has exactly the same shape with C₆₀. It should be noted, however, that E. Osawa predicted the presence of the spherical fullerene C₆₀ in 1970's. Because the results were published in a Japanese journal, respectively, the hypothesis did not reach the Western civilization.

Figure 1. Structures of a) Buckminster fullerene C60 [3], b) carbon nanotube [4], and c) carbon nanobud [5].

It follows from Euler's theorem that there are always exactly 12 pentagons in buckyballs, whereas the number of hexagons determines the size of the fullerene. Because the number of pentagons is constant, the smallest possible buckyball is C₂₀. Moreover, fullerenes with up to 100 carbon atoms are common. However, the C₆₀, which has a diameter of ca. seven angstroms, is the most common and therefore most studied fullerene. Even though all the carbons in the fullerene are conjugated, the fullerene molecule is not aromatic. Consequently, the electrons of the hexagonal rings of the fullerene do not delocalize over the whole molecule. The double bonds of the fullerene are not equal but the hexagon-hexagon bonds are shorter than the hexagon-pentagon bonds. [6]

Fullerenes are stable but not completely unreactive. The characteristic fullerene reaction is electrophilic addition to hexagon-hexagon double bonds. In the addition the sp² -hybridized carbons are changed into sp³ -hybridized ones. The change in hybridized orbitals decreases the bond angles and the bonds have to bend less when the spherical structure is formed and the fullerene becomes more stable. [6]

Fullerenes are hardly soluble to the polar solvents. However, fullerenes are the only allotrope of carbon that can be dissolved in a common solvent in a room temperature. The most common solvent that dissolves fullerenes is benzene. Solutions of pure C₆₀ and C₇₀ have deep purple and reddish brown color, respectively. [7]

It is possible to trap a metal atom inside the fullerene cage. These endohedral species are assigned as M@C₆₀, in which the M stands for metal. Also helium can be trapped inside the fullerene cage by heating fullerene in helium vapor under high pressure. [1] If the M is alkali metal, the structure of the M@C₆₀ is rather disordered. The bulk structure consists of grains, i.e. small regions of a crystal structure. The size of the grains may vary between tens of Angstroms to some microns. The orientation of the individual grains may not have any correlation at all. [8] The interaction between fullerene and alkali metals is based on the high electron affinity of the fullerene and electropositivity of the alkali metals.

Nowadays the development of organic solar cells is a hot topic. An essential task in developing the solar cell is to find an appropriate electron transfer pair. Porphyrin is an obvious chromophore to be used in the electron transfer dyads because of its occurrence in the photosynthetic reaction center in the nature. The quinone acceptor involved in the reaction center is, however, more often replaced with a fullerene. [9] The reason is simple, e.g. fullerene C₆₀ can accept up to six electrons. [10] The high electron affinity of the fullerene is due to its energetically low-lying triply degenerate lowest unoccupied molecular orbitals (LUMOs). [11] Nowadays there are plenty of investigations reporting porphyrin-fullerene dyads. An example of such a dyad is presented in Figure 2.

Figure 2. An example of a porphyrin-fullerene donor-acceptor dyad [9].

The poor water solubility of the fullerenes has restricted their biological applications. There has been, however, several attempts to try to use fullerenes e.g. in enzyme inhibition and antiviral activity. Most of these attempts date back, close to the discovery of the fullerenes, to the early 90's. [12] Although, the solubility of the fullerenes can be enhanced by adding surfactants, the carbon nanotubes are actually more promising than buckyballs as it comes to applications.

The nanotubes were officially discovered shortly after buckyballs, i.e. in 1991, by Iijima Sumio [13]. However, the history of the nanotubes is very similar to that of buckyballs. The nanotubes vere actually found almost 40 years before the work of Sumio as Radushkevich and Lukyanovich reported hollow graphitic fibers in 1950's in a Russian journal.

Nanotubes are cylindrical fullerenes which have a diameter of few nanometers and length of up to millimeters [14]. Straight (defectless) nanotubes have only hexagonal rings in their structure. If nanotubes have closed ends, they must contain also pentagonal rings (for the sake of Euler's theorem). Nanotubes may also contain heptagonal rings, which induce changes to the diameter of the nanotube. There are basically two types of nanotubes, single-walled (SWNT) and multi-walled nanotubes (MWNT). Double-walled nanotubes (DWNT) are a typical example of MWNTs.

The nanotubes have only sp² hybridized bonds and they are actually bent sheets of graphine. This explains the extraordinary strengths of carbon nanotubes. The nanotubes are actually stronger than diamond since diamond structure has weaker sp3 bonds. The properties of the nanotube depend how the graphite sheet has been rolled into a tube and therefore the nanotubes have been named on these basis. If integers n and m are the number of unit vectors of a graphine structure, the different types of nanotubes can be characterized by using these integers. Figure 3 illustrates the 'zigzag' (n, 0), 'armchair' (n,n) and 'chiral' (n,m) structures of nanotubes.

Figure 3. Forming of the zigzag, armchair, and chiral nanotube. [5]

The conductivity of an individual nanotube (n,m) depends on the helical orientation of the hexagonal rings in the walls of the nanotube i.e. on the n and m in a following way. If n and m are equal (the armchair structure), the nanotube is metallic and otherwise the nanotube is a semiconductor. Metallic nanotubes can carry over 1000 times higher electrical current densities than metals. Additionally, electrons will transport only along the direction of the axis of the nanotube. [15] There are almost limitless amount of possible applications of the conducting nantoube bundles. Electronic flat panel devises are just one example. [1] In addition to the electron conductance, nanotubes are also clearly better heat conductors than metals. [16]

Owing to their unique properties, there are several potential applications for carbon nanotubes. The electronic properties and size of nanotubes allow several applications in electronics. These include transistors that are capable for switching by using just one electron [17], or ultra light and efficient coolers for electronics [18]. The extreme strength / weight ratio of carbon nanotubes make them potential materials for armors [19]. These are just few examples of the diverse potential of the nanotubes.

Carbon nanobuds are molecules in which the spherical fullerene is covalently linked to the outer sidewall of a nanotube. Nanobuds combine properties of the buckyballs and nanotubes. For example, the conductivity of the nanobuds is similar to that of nanotubes, but nanobuds are more reactant than nanotubes because the attached buckyball. [20]

Two decades after the discovery of the fullerene family, the third fell-defined allotrope of carbon, the scientists have learned much about the fascinating properties of these molecules. Thought the chemistry of the fullerenes is yet far from being completely solved, it seems clear that these molecules have huge potential for applications in several different fields of science.

[1] H.W. Kroto, D.R.M. Walton, Fullerene. (2009). In Encyclopedia Britannica. Retrieved August 7, 2009, from Encyclopedia Britannica Online: http://search.eb.com/eb/article-234438

[2] H.W. Kroto, J.R. Heath, S.C. O'Brien, R.F. Curl, R.E. Smalley, Nature 318 (1985) 162.

[3] Nature.com, retrieved August 8, 2009 from: http://www.nature.com/nature/history/images/timeline/1980_6.jpg

[4] The International NanoScience community, retrieved August 8 2009 from: http://api.ning.com/files/hhEvnlUQK-zEz08mtquoJu3yTOoEIMYLTvZl5lvQzSDUwyfgwwXIw6LxNXnKDbXK8Qge6Hu4L7V-7XHsuPFp9cs*prT1NOwv/tube_angled.jpg

[5] Wikipedia.com, retrieved August 8 2009 from: http://commons.wikimedia.org/wiki/Category:Carbon_nanotube

[6] M. Prato, J. Mater. Chem. 1 (1997) 1097.

[7] V.N. Bezmelnitsyn, A.V. Eletskii, M.V. Okun, Russian Academy of Sciences, 41 (1998).

[8] K. Allen, F. Hellman, Phys. Rev. B 60 (1999) 11765.

[9] Q. Xie, E. Perez-Cordero, L. Echegoyen, J. Am. Chem. Soc. 114 (1992) 3978.

[10] R.C. Haddon, L.E. Brus, K. Raghavachari, Chem. Phys. Lett. 125 (1986) 459.

[11] P.A. Liddell, J.P. Sumida, A.N. MacPherson, L. Noss, G.R. Seely, K.N. Clark, A.L. Moore, T.A. Moore, D. Gust, Photochem. Photobiol. 60 (1994) 537.

[12] A.W. Jensen, S.R. Wilson, D.I. Schuster, Bioorg. Medic. Chem. 4 (1996) 767.

[13] S. Iijima, Nature, 354 (1991) 56.

[14] L.X Zheng, M.J. O'Connell, S.K. Doorn, X.Z. Liao, Y.H. Zhao, E.A. Akhadov, M.A. Hoffbauer, B.J. Roop, Q.X. Jia, R.C. Dye, D.E. Peterson, S.M. Huang, J. Liu, Y.T. Zhu, Nature Materials 3 (2004) 673.

[15] S. Hong, S. Myung, Nature Nanotechnology 2 (2007) 207.

[16] E. Thostenson, C. Li, T.-W. Chou, Composites Science and Technology 65 (2004) 491.

[17] Ch. Postma, T. Teepen, Z. Yao, M. Grifoni, C. Dekker, Science 293 (2001) 76.

[18] K. Kord's, G. T'th, P. Moilanen, M. Kumpum'ki, J. V'h'kangas, A. Uusim'ki, R. Vajtai, P.M. Ajayan, Appl. Phys. Lett. 90 (2007) 123105.

[19] T. Ylidrim, O. G'lseren, C. Kilic, S. Ciraci, Phys. Rev. B 62 (2000) 12648.

[20] A.G. Nasibulin, P.V. Pikhitsa, H. Jiang, D.P. Brown, A.V. Krashennikov, A.S. Anisimov, P. Queipo, A. Moisala, D. Gonzalez, G. Lientsching, A. Hassanien, S.D. Shandakov, G. Lolli, D.E. Resasco, M. Choi, D. Tomanek, E.I. Kauppinen, Nature Nanotechnology 2 (2006) 156.

High pressure Raman spectroscopy of double wall carbon nanotubes

Double wall carbon nanotubes are the simplest representation of multiwall carbon nanotubes. Higher electrical conductivity then single wall carbon nanotubes, existence of excitonic states and shielding of inner wall by outer wall to maintain the purity makes the double wall carbon nanotubes attractive for potential applications in the field of electronics and composite materials. In this essay we discuss the high pressure Raman spectroscopy of double wall carbon nanotubes to explore the properties of the walls of carbon nanotubes and the interaction between the walls of carbon nanotubes.

Raman spectroscopy has been very useful technique to characterize the structural properties carbon nanotubes. When a light wave, considered as a propagating oscillating dipole, passes over a molecule, it can interact and distort the cloud of electrons round the nuclei. As the oscillating dipole interacts with the molecule as it passes, it causes the electrons to polarize. The light is released immediately as scattered radiation. This process differs from an absorption process as the additional energy does not promote an electron to an excited state of the static molecule, in addition that the radiation is scattered as a sphere and not lost by energy transfer within the molecule or emitted at a lower energy. When scattering occurs, only a small fraction of the radiation energy is scattered. Mainly, the frequency of the scattered electromagnetic radiation is unchanged and the elastic scattered radiation gives rise to the so-called Rayleigh scattering. However, a smaller number of photons may scatter in-elastically; the interacting molecule can be excited from the ground state to any vibrational state; if this happens, the law of conservation of energy states that the scattered photons have energy slightly different from the frequency of the incident photons (Stokes scattering). It is also possible that an excited molecule will return from its excited state to its ground state and this process adds the energy of the transition to an incident photon, resulting in the shortening of the wavelengths of the scattered radiation (anti-Stokes scattering).

For high pressure experiments Diamond Anvil Cell (DAC) and Renishaw Raman spectrometer was used with laser wavelength 633 nm. The operation of the diamond anvil cell relies on the following principle: Pressure = Force / Area Therefore high pressure can be achieved by applying a moderate force on a small area, rather than applying a large force on a large area. In order to prevent deformation and even breakage of the anvils that apply the force, they must be made from a very hard and virtually incompressible material: Diamond. The standard DAC has been extensively used to the range of 10 - 300 kbar. Before high pressure Raman spectroscopy nanotubes were doped pressure transmitting medium and then sonicated. Gas kits to be used in DAC for high pressure were prepared. After that nanotubes were loaded in the DAC with ruby. Ruby lines are used to measure the pressure. Ruby lines shifts linearly with pressure.

Here the behavior of double walled carbon nanotubes with sulphuric acid as pressure transmitting medium under pressure through their higher energy first order Raman mode (GM) is discussed.

Inner and outer wall Raman shift with pressure. Using the differences in the GM line-shape and shift, the behaviour of the double wall carbon nanotubes prepared by different routes tubes under pressure is examined. The results suggest a blue shift in these transitions while increasing pressure. In addition, a variation in value of the transition pressure, when loading the different samples under same pressure range is observed. This variation was related to a variation in the diameter of the resonant tubes and difference of synthesis. The difference of slopes for inner and outer wall indicates the difference of interaction between the inner and outer tubes for different types of tubes. The difference of pressure experienced by same sample with the variation of concentration of sulphuric acid is also observed. This suggests the difference of structuring of sulphuric acid around the nanotubes when concentration is changed.

[1] P. Puech, A. Bassil, J. Gonzalez, Ch. Power, E. Flahaut, S. Barrau, Ph. Demont, C. Lacabanne, E. Perez, and W. S. Bacsa, Phys. Rev. B 72, 155436 (2005).

[2] Pascal Puech, Ahmad Ghandour, Andrei Sapelkin, Cyril Tinguely, Emmanuel Flahaut, David J. Dunstan, and Wolfgang Bacsa,1Phys. Rev. B 78, 045413 (2008)

[3] P. Puech, E. Flahaut, A. Bassil, T. Juffmann, F. Beuneu and W. S. Bacsa, J. Raman Spectrosc. 2007; 38: 714–720

Carbon nanotubes in solar cells and other energy applications

Introduction.

With the dawn of 21st century, increasing global energy needs and the environmental pollution have made renewable energy sources a hot topic for research. Breakthroughs in nanotechnology open up the possibility of moving beyond our current alternatives for energy supply by introducing technologies that are more efficient, inexpensive, and environmentally friendly. The integration of nanotechnology in energy has raised hopes among the researcher that it would dramatically help to reach the target. Carbon nanotubes are intensively being studied and employed in different energy technologies to increase the efficiency and to decrease the prices effectively. 85% of the today’s energy needs are fulfilled by fossil fuels which cause carbon emission in the atmosphere. So the importance of clean energy generation have become more important, specially the solar cells.

There are several kinds of solar cells. The 3rd generation solar cells are trying to produce cheaper electricity with improved efficiency with the help of CNTs. Dye sensitized nanostructured solar cells (DSCs) are photo electrochemical solar cells, consisting of a photoelectrode, redox electrolyte and counter electrode.

Fig 1: Structure of dye sensitized nanostructured solar cell [1]

Carbon nanotubes (CNTs) can be applied to the DSCs at photoelectrode, counter electrode and in electrolyte. Transport of electrons through the nanoporous film of TiO2 is sometimes problematic due to grain boundaries and increase in recombination. Due to the excellent electrical properties of CNTs (ballistic electron transport), it facilitates the electron transport and improves the short circuit current of the DSCs. The single wall CNTs (SWCNTs) seem to be quite adherent to TiO2 particles and cause TiO2 particles to aggregate. On the negative side there is less adsorbed dye and SWCNTs are non-uniformally distributed. There are some stability issues as well which need to be resolved for the practical application of CNTs at photoelectrode.

Acid treated multiwall CNTs (MWCNTs) are successfully employed at the photoelectrode of DSCs at low temperature (sintering at 150oC for 4 hours).

CNTs form gels with ionic liquids by grinding which improves the transport properties in the electrolyte [4]. When MWNTs and SWNTs are dispersed into ionic liquid electrolytes, an improvement in fill factor and open circuit voltage is observed [5].

MWCNT counter electrodes were found to be more efficient and stable than sputtered Pt and electro-deposited Pt counter electrodes in a 5 day stability test at room temperature [6]. In a short term i.e. 30 days stability test in dark at room temperature for MWCNTs, a continuous decrease in short circuit current density Isc was observed due to detachment of weakly adheared CNTs from the FTO glass and deposition on photoanode side [7]. The larger diameter CNTs show better performance [7]. A short term stability of SWCNTs for 12 days at room temperature was reported [8]. A composite film of grapheme (1%wt) and PEDOT-PSS showed significantly improved performance as counter electrode on ITO substrates [9].

CNTs in DSC components seem to improve the performance of the DSCs. However in literature amounts / weight percentages are announced carelessly. CNT incorporation and sorting is difficult, and manipulation methods may need to be improved further. CNTs are still rather expensive (even though one does not need much in composites), that is why synthesis methods in large quantities should be developed.

Besides various promising application of CNTs in photovoltaics technology, there has also been advances in the use of CNTs to improve energy efficiency of wind turbines as an alternative renewable energy technology. For example, Eagle Windpower Oy, a Finnish technology company, has developed a solution to utilize CNTs and epoxy that binds nanotubes to make the windmill blades stronger and lighter (similar manufacturing technology was utilized in e.g. ski manufacture). In comparison with conventional glassfiber blades, the CNT-reinforced blades are 50% lighter, which allows the increase of the blade size, and extremely durable. Such small wind power stations are becoming more and more widespread nowadays as a simplified solution of locally produced wind power in houses, leisure homes and in agriculture [10].

Furthermore, CNTs were utilized to fabricate high power supercapacitors- electrical storage devices that are able to deliver a large amount of energy in a short period of time. For example, MIT researchers are on the way to commercialize a battery based on capacitors that use CNTs for high surface area, allowing fast charging and no degradation being advantageous over traditional batteries that use a chemical reaction to produce energy [16]. Recently, MIT researchers have demonstrated a layer-by-layer method to fabricate pure, dense, thin films made of uniform CNT layers able to carry and store a large amount of charge, making them promising electrode materials [12].

Fig 5: Scanning-electron-microscope image of a film made up of 30 layers of the nanotubes on a silicone substrate [12]

Figure 5 (B) is an example of a simple structure of a supercapacitor using a dense CNT network fulfilling two main functions in one single layer: an electrically conducting material for the replacement of the metallic current collector, and the active material to build up the electrochemical double layer. Such a charge storage device is lightweight, low-cost and requires simple room-temperature manufacturing technique [13].

Fig 6: Sketch (left) and picture (right) of a supercapacitor using CNT networks for both the current collector and the electrode. The CNT network and the separator (white strip) are encapsulated in a PET container filled with electrolyte [13].

Recently, a new CNT-based solution was proposed to reduce the costs of fuel cells where traditionally expensive platinum catalysts are used, which also has other problems like reduced efficiency due to surface-adhered carbon monoxide and properties degradation with time. L. Dai’s group has published in Science a novel idea of using an array of vertically aligned nitrogen-containing carbon nanotubes (VA-NCNTs) produced by pyrolysis of iron(II) phthalocyanine in either the presence or absence of additional NH3 vapor, that could be used as an oxygen reduction reaction (ORR) electrocatalysts even after the removal of the residual Fe catalyst, showing a better long-term operation stability than similar platinum-based electrodes [14].

Fig 7: (A) SEM image of the as-synthesized VA-NCNTs on a quartz substrate. (B) TEM image of the electrochemically purified vertically aligned nitrogen-containing carbon nanotubes (VA-NCNTs). (C) Digital photograph of the VA-NCNT array after having been transferred onto a PS-nonaligned CNT conductive nano-composite film. Scale bars, 2 µm (A); 50 nm (B) [14].

According to European Union Photovoltaic Platform, 15% of the world’s electricity will be from solar by the year 2050. It shows that PV sector has a bright future. The integration of CNTs in DSCs is drastically improving the performance of dye sensitized solar cells. CNTs have also been employed successfully in other renewable energy sources like wind mill, hydrogen storage etc. Moreover, CNTs can have wide applications as alternative energy storage media, utilizing fully their unique structural characteristics.

[1] http://research.ncku.edu.tw/re/articles/e/20080516/5.html

[2] Kongkanand et al., Nano Lett. 7 (2006) 676680

[3] Lee et al., Sol. Energy Mater. Sol. Cells (2008) Sawatsuk et al., Diamond & Relater Materials 18(2009), 524

[4] Science 300, 2072 (2003)

[5] Usui et al., J. Photochem. Photobiol. A: Chem. 164 (2004), 97101

[6] B.K. Koo, D.Y. Lee, H.J. Kim, W.J. Lee, J.S. Song, and H.J. Kim“Seasoning effect of dye-sensitized solar cells with different counter electrodes", J. Electroceram (2006) 17:79-82. DOI: 10.1007/s10832-006-9941-x

[7] T. N. Murakami, S. Ito, Q. wang, M.K. Nazeeruddin, T. Bessho, I. Cesar, P. Liska, R. Humphry-Baker, P. Comte, P. Pechy, and M. Grätzel,"Highly efficient dye sensitized solar cells based on carbon black counter electrodes", Journal of The Electrochemical Society, 153 (12) A2255-A2261 (2006).

[8] H. Zhu, j. Wei, K. wang, and D. Wu, “Applications of carbon materials in photovoltaic solar cells", Solar Energy Materials and Solar Cells .Volume 93, Issue 9, September 2009, Pages 1461-1470. doi:10.1016/j.solmat.2009.04.006

[9] P. Joshi, Y. Xie, M. Ropp, D. Galipeau, S. Bailey, and Q. Qiao, “Dye-sensitized solar cells based on low cost nanoscale carbon/TiO2 composite counter electrode" Energy Environ. Sci., 2009, 2, 426-429. DOI: 10.1039/b815947p

[10] [Advanced solution for harnessing light winds, Energy and Enviro, TechKnowledge Ltd, 2009: www.energy-enviro.fi].

[11] [Nanotube Superbatteries, K. Bourzac, Technology review by MIT, Jan. 2009]

[12] Bifunctional carbon nanotube networks for supercapacitors, M. Kaempgen, J. Ma, G. Gruner, G. Wee and S. G. Mhaisalkar, Applied Phys Lett, 90, 2007].

[13] Nitrogen-doped carbon nanotube arrays with high electrocatalytic activity for oxygen reduction, K.Gong, F.Du, Z.Xia, M.Durstock, L.Dai, Science, Vol. 323, No 5915, pp. 760-764, 2009.

[14] Wikipedia

Plasmon coupling in carbon nanotubes

Single-walled carbon nanotubes (CNT) are quasi-one-dimensional (1D) wires formed by graphene sheets rolled-up into cylinders with diameters 1-10nm and lengths 1-104 µm [1].

Each nanotube with particular (n,m) indices have characteristic electronic properties. The electronic density of states is a “fingerprint� of particular nanotube. In general carbon nanotubes can have metallic or semiconducting properties (the gap is either absent or it is present, see fig. 1) [2].

There are several mechanisms of light interaction with a nanotube: absorption, scattering, emission, diffraction and surface plasmon resonance (coherent oscillation of conductance electrons).

When light interacts with dielectric or semiconductor the creation of exciton (a bound state of electron and hole) occurs. The predominant part of optical experiments is done with semiconducting nanotubes. So, The theory was developed to research the possibility of exciton-plasmon coupling in semiconducting carbon nanotubes. The tube is modeled as infinitely thin, infinitely long, anisotropically conducting cylinder with its surface conductivity ( is taken into account) obtained from the realistic band structure of a particular CNT. The total Hamiltonian of the coupled exciton-photon system on the nanotube surface is of the form:

(1)

The first term represents free (medium assisted) electromagnetic (EM) field. It is possible to use the second quantized field Hamiltonian, which accounts the creation and annihilation of surface EM excitation of frequency at a point associated with carbon atom ( or n describes the position of the atom). The second term represents free exiton. The second quantized field Hamiltonian accounts the creation and annihilation of exiton with energy , here index f describes internal degrees of freedom of exiton. And the third term represents interaction between EM field and exiton. The physical meaning of the third term is following the exciton with the energy is excited through the electric dipole transition in the lattice site by the transversely (longitudinally) polarized surface EM modes.

This theory predicts that, first of all, the strong exciton-plasmon coupling in semiconducting carbon nanotubes must occur. In this energy region the dispersion curve of semiconducting carbon nanotube is continuous curve. But, when plasmon coupling occurs, the exciton dispersion curve exhibits Rabi-splitting (fig.2) [1]. If the dispersion curve changes so dramatically, the conductance also changes, when the plasmon coupling occurs. This can have variety of applications in the field of nanoelectronics and photonics. Such device as surface plasmon polariton controlled carbon nanotube transistor can be made.

[1] I.V. Bondarev, K. Tatur, L.M. Woods. Optics Communications. 282 (2009)

[2] R.Saito, M.Fujita, G.Dresselhaus, and M.S. Dresselhaus. Appl.Phys.Lett., vol.60 (1992).

Magnetic behavior of carbon nanosturtures: theoretical understanding and experimental controversies

At present time structures made up atoms of carbon that have nanometer scales became very interesting objects of investigation in spheres of condensed state physics and inorganic chemistry. Among these structures one can point out first of all, fullerenes, carbon nanotubes and thin multilayered nanostructures. They are interesting due to their unusual physical parameters (mechanical, electromagnetical and so on) that are absolutely absent in macro-material structures consistent of the same atoms. To explain the phenomena connected with displaying of such outstanding physical parameters one can apply laws of quantum physics, because changing ordinary properties of carbon starts after decreasing a size of examined structure until value ~ 100 nm. Carbon nanostructures are interesting both for studying fundamental properties of matter and for development of new informational and computers’ devices, for example sensing heads, elements of computer memory, etc.

As chemical element, carbon is a component of a lot of natural and synthetic materials thus, in macro-world it is well-known diamagnetic, in spite of that carbon nanostructures have unusual magnetic properties. Anybody knows that carbon in the form of graphite has enough high value of diamagnetic susceptibility χ~R², here R is radius of circulated current ( for π-electrons, that cover 36 fundamental units of graphite, R≈ 0.78 nm). If an external constant magnetic field is oriented perpendicularly to graphite’s planes then the χ value for graphite is 25 times larger than the diamond’s one. But for carbon nanotubes immersed into an external magnetic field that is oriented parallel to the tubes axes circulation of electrons take place along their surfaces thus R≈ 8 nm, therefore their diamagnetic susceptibility value is hundred times much as compared with value of this parameter for graphite.

Thin films made up of fullerens C₆₀ illustrate untypical for macro-samples of carbon materials magnetic behavior in the Earth's magnetic field influenced by polarized light. Two samples of 30 nm and 250 nm thickness have demonstrated a significant changing of magnetic field intensity, in range from 3.4 to 12.9 nT, under the influence of polarization light.

Magnetic behavior of carbon nanostructures strongly depends upon impurity atoms either inserted into inner space of fullerens or embedded into a nanotube’s carcass instead of some carbons’ atoms. In the capacity of such admixture one can mainly utilize atoms of irons’ group: Fe, Co and Ni. Development of air-stable single magnetic domain room temperature magnets is an important goal of nanotechnology. Recent important progress in stabilizing cobalt nanomagnets has been made through carbon encapsulation of the metal particles. The structures exhibit the magnetic hysteresis curve with a coercive force of H ≈ 200 Oe at low temperatures T~20 K and 260 Oe at 300 K. The temperature dependence of the magnetic susceptibility measured under zero-field cooling and 10 Oe field cooling conditions exhibits the behavior characteristic of a set of single magnetic domain nanomagnets in an amorphous carbon matrix. Single magnetic domain particles are expected to show weak temperature dependence of the coercive force, while the saturation magnetization of the single domain magnets increases. Single domain magnets in carbon matrix are also expected to interact with each other through magnetic dipole-dipole interaction minimizing the global magnetization of the system at zero magnetic fields. In hetero-structured carbon nanotubes, partly filled by impurities on interface of carbon carcass and boron nitride segments a constant magnetic moment can acquire. Depending on the atomic arrangement, artificially formed super-lattices exhibit an itinerant ferromagnetic behavior. In some other nanostructures, presence of carbon radicals can lead to ferromagnetic behavior with a high Curie temperature. Unexpected giant magneto-conductance occurs in twisted nanotubes in presence of an external magnetic field.

Striking magnetic behavior has been found out in clusters of nanotubes, that compose general column with common outer surface (such structures at present time can be obtained during plasma discharges). If this nanocolumn is effected by an external magnetic field orielted perpendicularly to its axis of symmetry then magnetic flow of each tube becomes captured by the external field that induces electric currents which can circulate during some hours under the Helium temperatures. Even under the room temperatures this current is weakly damped: in some experiments value of eigen magnetic moment decreased half as much during two hours at T≈100°Ñ. It means that electric current in nanostructures is characterized by abnormally high level of electric conductivity as compared with its value in conductors made up of macro materials. Theoretical investigation of this phenomenon proved that it can be explained only by application of laws of quantum physics, because through nanocolumns with diameter 20 nm and length 10^-5m under room temperatures one can transmit electric current with density j≈10⁷A/cm² and quantity of heating power will be only ~0.003 W. Therefore such high level of electric conductivity in nanocolumns exhibits its quantum character.

Concerning theoretical understanding of electromagnetic properties of nanostructures I can say that it keeps up with their experimental study barely. It’s connected at first, with abstractness and complicated form of quantum physics laws; second, carrying out theoretical investigation of these properties one can be aware of multifunctional character of magnetic behavior’s dependence both upon physical parameters of the studied nanostructure and upon manner of their production, presence of impurities and defects in carbon’s carcass; and at least, with technical difficulty of experiments, including preparation nanostructures. So in my mind, now the role of theoreticians in research into properties of nanostructures is auxiliary one, they only gather data, experimental facts, try to explain some dependences and experiments.

Electronic structure and properties of graphene

Graphene is made from carbon atoms arranged on a honeycomb crystall lattice made out of hexagons (see the Fig.1 [1]). One can call the graphene as a one-atom-thick planar sheet of sp2-bonded carbon atoms that are densely packed in a honeycomb structure.

Electronic structure of the graphene is based on the sp2 hybrids in which hybrid orbitals will form only σ bonds and one π (pi) bond is needed for the double bond between the carbons [2]. In this hybridization (mixing atomic orbitals) the 2s orbital is mixed with only two of the three available 2p orbitals [3] [4] [5]. The carbon-carbon bond length in graphene is approximately 0.142 nm. Graphene appears the basic structural element of some carbon allotropes including graphite, carbon nanotubes and fullerenes.

Concerning electronic properties of the graphene, it can be a semi-metal or zero-gap semiconductor. The dispersion relation between energy and wave number is linear for low energies near the six corners of the two-dimensional hexagonal Brillouin zone, leading to zero effective mass for electrons and holes [6] [7] yielding that at such low energies, electrons and holes near these six points, two of which are inequivalent, behave like relativistic particles described by the Dirac equation for spin 1/2 particles [8] [9] and the electrons and holes are called as DIrac fermions, and the corresponding six corners are Dirac points .

Experimentally it was observed that due to having unique electronic properties the graphene produces an unexpectedly high opacity for an atomic monolayer, with the absorbtion πα ≈ 2.3% of white light, where α is the fine-structure constant [10] [11]. Additionally, it has been shown that bandgap of graphene can be controlled from 0 to 0.25 eV by means of applying voltage to a dual-gate bilayer graphene field-effect transistor (FET) at room temperature [12]. Furthermore it was demonstrated that such unique absorption could become saturated ( non-linear behaviour called as Saturable absorption )when the input optical intensity above a threshold value (saturation fluency) giving opportunity for the graphene to be used in the wide application in ultrafast photonics because of ability of the graphene to be saturated under strong excitation from visible to near-infrared region due to the universal optical absorption and zero band gap. Also, the graphene can be utilized in spintronics as ideal material due to small spin-orbit interaction and near absence of nuclear magnetic moments in carbon, as well as electrical spin-current injection and detection in graphene was observed up to room temperature [13] [14] leading to such phenomena as spin coherence length above 1 micron at room temperature [15], and control of the spin current polarity with an electrical gate at low temperature [16].

Thermal conduction of the graphene is phonon-dominated [17]. Only in the case of a gated graphene strip the electronic contribution dominates over the phonon contribution at low temperatures when applying bias causing a Fermi Energy shift lareger than kT [18]. Despite its 2-D nature, graphene has 3 acoustic phonon modes. The two in-plane modes have a linear dispersion relation, whereas the out of plane mode has a quadratic dispersion relation. And the ballistic thermal conductance of graphene is isotropic [19].

Graphene is the strongest material ever tested [20] and thus, the graphine is very strong and rigid. For this reason the graphene can be utilized for NEMS applications such as pressure sensors, and resonators [21].

References:

[1] http://www.newworldencyclopedia.org/entry/Graphene

[2] J. P. Clayden, N. Greeves, S. G. Warren, P. D. Wothers (2000), Organic Chemistry (1st ed.), Oxford: Oxford University Press, p. 105, ISBN 978-0-19-8503 46-0

[3] Organic Chemistry, Third Edition Marye Anne Fox James K. Whitesell 2003 ISBN 978-0-7637-3586-9

[4] Organic Chemistry 3rd Ed. 2001 Paula Yurkanis Bruice ISBN 0-13-017858-6

[5] L. Pauling, J. Am. Chem. Soc. 53 (1931), 1367

[6] P. R. Wallace (1947). Physical Review The Band Theory of Graphite. 71. p. 622-634. http://link.aps.org/doi/10.1103/PhysRev.71.622 Physical Review.

[7] J.-C. Charlier, P.C. Eklund, J. Zhu, and A.C. Ferrari (2008). "Electron and Phonon Properties of Graphene: Their Relationship with Carbon Nanotubes". from Carbon Nanotubes: Advanced Topics in the Synthesis, Structure, Properties and Applications, Ed. A. Jorio, G. Dresselhaus, and M.S. Dresselhaus. Berlin/Heidelberg: Springer-Verlag.

[8] Semenoff, G. W. (1984). "Condensed-Matter Simulation of a Three-Dimensional Anomaly". Physical Review Letters 53: 5449. http://prola.aps.org/abstract/PRL/v53/i26/p2449_1.

[9] Avouris, P., Chen, Z., and Perebeinos, V. (2007). "Carbon-based electronics". Nature Nanotechnology 2: 605. http://www.nature.com/nnano/journal/v2/n10/abs/nnano.2007.300.html.

[10] Kuzmenko, A. B.; van Heumen, E.; Carbone, F.; van der Marel, D. (2008). "Universal infrared conductance of graphite". Phys. Rev. Lett. 100: 117401. doi:10.1103/PhysRevLett.100.117401.

[11] Nair, R. R.; Blake, P.; Grigorenko, A. N.; Novoselov, K. S.; Booth, T. J.; Stauber, T.; Peres, N. M. R.; Geim, A. K. (2008). "Fine Structure Constant Defines Visual Transparency of Graphene". Science 320: 1308. doi:10.1126/science.1156965. PMID 18388259. http://onnes.ph.man.ac.uk/nano/Publications/Science_2008fsc.pdf.

[12] Y. Zhang et al. (11 June 2009). "Direct observation of a widely tunable bandgap in bilayer graphene". Nature 459: 820-823. doi:10.1038/nature08105.

[13] Cho, Sungjae; Yung-Fu Chen, and Michael S. Fuhrer (2007). "Gate-tunable Graphene Spin Valve". Applied Physics Letters 91: 123105. doi:10.1063/1.2784934.

[14] Ohishi, Megumi; et al. (2007). "Spin Injection into a Graphene Thin Film at Room Temperature". Jpn. J. Appl. Phys. 46: L605-L607. doi:10.1143/JJAP.46.L605.

[15] Tombros, Nikolaos; et al. (2007). "Electronic spin transport and spin precession in single graphene layers at room temperature" (PDF). Nature 448: 571-575. doi:10.1038/nature06037.

[16] Cho, Sungjae; Yung-Fu Chen, and Michael S. Fuhrer (2007). "Gate-tunable Graphene Spin Valve". Applied Physics Letters 91: 123105. doi:10.1063/1.2784934.

[17] Balandin, A.A., Ghosh, S., Bao, W., Calizo, I., Teweldebrahn, D., Miao, F., and Lau, C.N. (2008). "Superior Thermal Conductivity of Single-Layer Graphene". Nano Letters ASAP. doi:10.1021/nl0731872.

[18] Saito, K., Nakamura, J., and Natori, A. (2007). "Ballistic thermal conductance of a graphene sheet". Physical Review B 76: 115409. doi:10.1103/PhysRevB.76.115409. http://link.aps.org/doi/10.1103/PhysRevB.76.115409.

[19] Saito, K., Nakamura, J., and Natori, A. (2007). "Ballistic thermal conductance of a graphene sheet". Physical Review B 76: 115409. doi:10.1103/PhysRevB.76.115409. http://link.aps.org/doi/10.1103/PhysRevB.76.115409.

[20] C. Lee et al. (2008). "Measurement of the Elastic Properties and Intrinsic Strength of Monolayer Graphene". Science 321: 385. doi:10.1126/science.1157996. http://www.sciencemag.org/cgi/content/abstract/321/5887/385. Lay summary.

[21] Frank, I. W., Tanenbaum, D. M., Van Der Zande, A.M., and McEuen, P. L. (2007). "Mechanical properties of suspended graphene sheets". J. Vac. Sci. Technol. B 25: 2558-2561. http://www.lassp.cornell.edu/lassp_data/mceuen/homepage/Publications/JVSTB_Pushing_Graphene.pdf.

Quantum transport in carbon nanotubes and graphene nanoribbons

Carbon nanotubes exhibit unusual physical and electronic properties what makes them extremely prospective for various applications in nanoscience, especially in nanotechnology. They can be metallic or semiconducting depending on their topology at the same time being mechanically strong - 100 times stronger though six times lighter than steel. All these properties together with high mobility of carriers in nanotube allow their utilization in nanocircuits as nanowires as well as building blocks in nano logic devices.

At the scale where the sizes (for example, the size of the wire) become small compared to the characteristic length of the electron motion, classical physics is not valid any more to describe physical phenomena. Instead, quantum effects appear. Consequently, electronic transport of nanotubes is also governed by the laws of quantum physics:In bulk materials according to Ohm’s law resistance depends on the material of the sample and its geometry: R = ρ L / A, where ρ is resistivity depending on the material only, L – the length of the piece of material and A - its cross sectional area. Unlikely, in nanotubes, ρ does depend on L through quantum effects which will be described below.

Typical construction for observing quantum transport in nanotubes is two electrodes connected by a nanonwire (nanotube). Two main regimes of transports can be met in nanotubes: diffusive and ballistic, depending on their type, whether they are single-walled metallic (SWM), single-walled semiconducting (SWS) or multiwalled (MW): Ballistic transport is encountered in SWM and MW carbon nanotubes. As for diffusive transport, it can be met in all the three types.

In diffusive regime electron undergoes (multiple) reflections caused by elastic scattering from impurities, therefore, electron wavefunction changes during electron motion. In this case mean free path (λ) is considerably smaller than width (W) and the length of the wire (L): λ << W,L, where λ=vFτ. Here, vF is the Fermi velocity and τ is the scattering relaxation time.

Ballistic transport is the case of an ideal conduction i.e. almost without scattering. As a result, the direction of the electron motion and consequently, its wavefunction remains unchanged. In this case λ >> W,L.

An amazing phenomena takes place in ballistic conductors at very low temperatures for the samples with small number of channels: contact conductance (as a result, resistance) is independent of material and size of the specimen, even more, is quantized in units of e2/h [1], each 1D channel conducts exactly 2e²/h kΩ. In case of multiple channels conductance is proportional to the number of channels determined by Landauer formula : G=(2e²/h)MT, M-number of channels, T transmission probability for the channel placed between two electrodes. The value of h/e² = 25kΩ is called the quantum of resistance. It must be emphasized that G is due to the contacts which is linked by the nanowire and not due to the wire itself.

Graphene nanoribbons (GNR) are unrolled sheets of single-walled carbon nanotubes. Like other carbon nanostructures they exhibit interesting electronic properties and is seen as an alternative to silicon in nanoelectronics Several studies using tight binding method have predicted that armchair GNRs can be either metallic or semiconducting depending on their widths [2-8]whereas ribbons with zigzag shaped edges are metallic [2-12].However, calculations through Density Functional Theory within Local Density approximation show that armchair nanoribbons can be semiconducting [13].

Experiments have shown that graphene nanoribbons have an energy gap near the charge neutrality point which decreases with increasing the width of the nanoribbon [14]. Explanation to the existance of an energy gap in nanoribbons with disordered edges has been suggested through Coulumb blockade [15] phenomenon, which arises due to the roughness at the edges of nanoribbons . Theoretical studies have shown that transport effects such as Coulomb blockade or a mobility gap caused by edge disorder [16,17] may affect the accuracy of bandgaps measured under transport conditions and explain the independence of energy gap and crystallographic orientation. However, latest experimental studies have revealed that the crystallographic orientation of the graphene edges significantly influences the electronic properties of nanoribbons [18].

It is notable that changing external conditions like doping, defects, chemical functionalization also may affect electronic properties of carbon nanostructures what can be used for tuning transport processes.

[1] B.J. van Wees et al., Phys.Rev.Lett (1988)

[2] M. Fujita, K. Wakabayashi, K. Nakada, and K. Kusakabe, J. Phys. Soc. Jpn. (1996).

[3] K. Nakada, M. Fujita, G. Dresselhaus, and M. S. Dresselhaus, Phys. Rev. (1996).

[4] K. Wakabayashi, M. Fujita, H. Ajiki, and M. Sigrist, Phys. Rev. (1999).

[5] M. Ezawa, Phys. Rev. (2006).

[6] L. Brey and H. A. Fertig, Phys. Rev. (2006).

[7] K.-I. Sasaki, S. Murakami, and R. Saito, J. Phys. Soc. Jpn. (2006).

[8] D. A. Abanin, P. A. Lee, and L. S. Levitov, Phys. Rev. Lett. (2006).

[9] S. Okada and A. Oshiyama, Phys. Rev. Lett. (2001).

[10] H. Lee et al., Phys. Rev. (2005).

[11] Y.-W. Son, M. L. Cohen, and S. G. Louie, Nature (London) (2006).

[12] Y. Miyamoto, K. Nakada, and M. Fujita, Phys. Rev. (1999)

[13] V. Barone, O. Hod, and G.E. Scuseria, Nano Lett. (2006)

[14] M.Y. Han, B. Özyilmaz, Y. Zhang, and P. Kim, Phys. Rev. Lett. (2007)

[15] F. Sols, F. Guinea, and A. H. Castro Neto, Phys. Rev. Lett. (2007).

[16] D.Querlioz, et al. Appl. Phys. Lett. (2008).

[17] D.Gunlycke, D. A. Areshkin, and C.T. White, Appl. Phys. Lett. (2007).

[18] Kyle A. Ritter and JosephW. Lyding, Nature Materials (2009)

Optical response of carbon nanostructures (nanotubes, fullerenes, graphene)

Carbon-based nanostructures are promising candidates for sensors and components in nanoscale circuitry [1,2]. Yet even with novel experimental geometries [3] and high-resolution scanning-probe techniques [4], transport measurements of individual molecules continues to be exceedingly difficult. Additionally, while recent measurements of the optical properties of single-walled carbon nanotubes (SWCNTs) have generated significant new insight [5,6], they have also raised major new questions about the role of many-electron effects in their absorption and luminescence spectra.

Stretching a carbon nanotube composite like taffy, researchers at the National Institute of Standards and Technology (NIST) and the Rochester Institute of Technology (RIT) have made some of the first measurements1 of how single-walled carbon nanotubes (SWNTs) both scatter and absorb polarized light, a key optical and electronic property. Recent research on the optics of SWNTs has focused on the behavior of “excitons" — the pairing of a negatively charged electron with the positively charged “hole" that it leaves behind when it gets excited by a photon into a higher energy state. An important optical characteristic is how excitons in SWNTs impact the way the nanotubes absorb and scatter light.For example, how easy is it for the incident light to deform an exciton to create positive and negative poles? Theory says it should be significantly harder to do in a nearly one-dimensional nanotube than in a bulk semiconductor, where nearby electrons and holes reduce the amount of energy required. Measuring that is difficult because the effect depends on the orientation of the nanotubes, and they’re hard to line up neatly. The NIST/RIT team solved the problem elegantly by wrapping SWNTs with DNA to keep them from clumping together, and dispersing them in a polymer. When they heated the polymer and stretched it in one direction, the nanotubes aligned like sugar crystals lining up in pulled taffy, making the optical measurements possible. The team obtained the first experimental verification of the full optical response of individual semiconducting SWNTs, finding good agreement with theory.

In (8) was showed two new developments for treating the properties of nanostructured systems beyond their ground states. First, we outlined a new scattering-state approach for computing transport properties at finite bias voltage; then we discussed a many-electron Green’s function technique for accurate computation of optical excitations. We considered two examples, four-atom carbon atomic wire nanojunctions and single-walled carbon nanotubes. For C4 nanojunctions, we predicted and explained the origin of the negative differential resistance and illustrated the important role the contact plays in determining the I –V characteristics of molecular junctions. For carbon nanotubes, we showed that in order to explain qualitatively and quantitatively the optical absorption spectra of these carbon nanostructures, it is crucial to account formany-body (excitonic) effects beyond the conventional, one-particle level.

In (9) was showed through the DFWM measurements that the fullerene series from C60 to C96 shows a large thirdorder nonlinear optical response at 0.532 ím. The concentrations of fullerenes and the incident laser powers were found to be vitally important in the DFWM measurements and are therefore optimized. The second hyperpolarizabilities of these fullerenes were determined to be on the order of 10-31-10-30 esu, in which exists a trend that higher fullerenes show larger ç1111. The reasons for the trend were discussed based on the model of a free-electron gas confined in a spherical shell and the distortion-enhanced and resonance-enhanced third-order optical nonlinearity.

It is well known that nanophotonics problems show the new ways to study organic materials doped with fullerenes. A fullerene introduction in these materials is widely used due to high electron affinity of fullerenes (2.6–2.7 eV) that allows the intramolecular donor–acceptor interaction to be reinforced. As it has been demonstrated in papers [10-11] for different donor–acceptor structures, electron transfers not to the intramolecular acceptor fragment but to fullerene one. This complex is of a higher excited state absorption cross-section than the ground state one, it reveals unique spectral and dynamic characteristics

The optical response of gapped graphene is of importance for several reasons. First, it is needed for an understanding of optoelectronic devices such as photodetectors and lightemitting devices. Second, optical spectroscopy might be applied for measurements of the magnitude of the energy gap. Graphene is an appropriate system to examine because it is a two-dimensional semimetal [12-14], which has generated considerable interest because of the interesting physics associated with its unusual electronic structure and its promising device applications [15]. In particular, the optical properties of graphene display many intriguing features, such as a constant optical conductivity in the infrared-frequency regime, gatedependence optical absorbance, and a unique magneto-optical spectrum [16-18].

[1] C. Dekker, Phys. Today 52 (1999) 22.

[2] H. Park, J. Park, A.K.L. Lim, et al., Nature 407 (2000) 57.

[3] M.A. Reed, C. Zhou, C.J. Muller, et al., Science 278 (1997) 252.

[4] B.Q. Xu, N.J. Tao, Science 301 (2003) 1221.

[5] Z.M. Li, et al., Phys. Rev. Lett. 87 (2001) 127401.

[6] S.M. Bachilo, et al., Science 298 (2002) 2361.

[7] J.A. Fagan, J.R. Simpson, B.J. Landi, L.J. Richter, I.Mandelbaum, V. Bajpai, D.L. Ho, R. Raffaelle, A.R. Hight Walker, B.J. Bauer and E.K. Hobbie. Dielectric response of aligned semiconducting single-wall nanotubes. Physical Review Letters. 98, 147402 (2007).

[8] Electron transport and optical properties of carbon nanostructures from first principles J.B. Neaton et al. / Computer Physics Communications 169 (2005) 1–8

[9] Third-Order Nonlinear Optical Response of Fullerenes as a Function of the Carbon Cage Size (C60 to C96) at 0.532 í Huang et al. J. Phys. Chem. B 1998, 102, 61-66 [10] N.V. Kamanina, V.S. Vikhnin, A. Leyderman, A. Barrientos, Y. Cui, M. Vlasse, Opt. Spectrosc. 89 (2000) 369. [11] N.V. Kamanina, Synth. Met. 127 (2002) 121.

[12] K. S. Novoselov et al., Science 306, 666 (2004).

[13] K. S. Novoselov et al., Nature (London) 438, 197 (2005).

[14] Y. Zhang, Y.-W. Tan, H. L. Stormer, P. Kim, Nature 438, 201 (2005).

[15] A. K. Geim and K. S. Novoselov, Nature Materials 6, 183 (2007); A. H. Castro Neto, et al., Rev. Mod. Phys. 81, 109 (2009), and references therein.

[16] F. Wang, et al., Science 320, 206 (2008).

[17] D. S. L. Abergel and Vladimir I. Fal'ko, Rev. B 75, 155430, (2007).

[18] V.P. Gusynin and S.G. Sharapov, Phys. Rev. B 73, 245411, (2006).

Carbon nanostructures beyond fullerenes, nanotubes and graphene: From nanobuds to foams to schwarzite to diamondoids

Carbon nanobuds form a material which combines two allotropes of carbon: carbon nanotubes and fullerenes, shown in Fig. 1. In this material fullerenes are covalently bonded to the outer sidewalls of the underlying nanotube. Therefore, nanobuds exhibit proberties of both carbon nanotubes and fullerenes. For example, the electrical conductivity and the mechanical properties of the nanobuds are similar to those of corresponding nanotubes. However, because of the higher reactivity of the attached fullerene molecules, the hybrid material can be further functionalized through known fullerene chemistry. [1]

Figure 1. Carbon nanobud form a material which combines two allotropes of carbon: carbon nanotube and fullerene [1]

Another interesting route to form low mass density all-carbon structures, but with significantly higher mechanical stability, are carbon foams, which may occur for example as honeycomb graphite structures (see Fig. 2), but can also expose nanopores of different geometrical shape. Their mechanical and electronic properties will be highlighted in this contribution. [2]

Figure 2. Structure of carbon foam (honeycomb graphite) [3]

One type of negatively curved periodical nanostructures was proposed by Terrones [4]. In the form of carbon zeolite-like structures; he called them Schwarzites (see Fig. 3). They appear to be some kind of cubic lattice, but they are two-dimensional structures and thus their properties differ from those we have in the ordinary crystalline materials. The carbon rings on the negative curvature surfaces of schwarzite structures are expected to include heptagons and/or octagons in addition to an indeterminate number of hexagons. The carbon heptagons and octagons introduce negative curvature into the schwarzite surfaces. [4]

Figure 3. The four cells of Schwarzite [4]

In Fig. 3 diamondoid refers to variants of the carbon cage molecule known as adamantane (C10H16), the smallest unit cage structure of the diamond crystal lattice [5]. The unique structure of adamantane is reflected in its highly unusual physical and chemical properties. The adamantane comprises a small cage structure. Because of this, adamantane and diamondoids in general are commonly known as cage hydrocarbons. In a broader sense they may be described as saturated, polycyclic, cage-like hydrocarbons that are present in some reservoir fluids. [5]

Figure 4. The smallest unit cage structure of the diamond crystal lattice is known as adamantane [5]

[1] Nasibulin, A. G. et al. Nature Nanotechnology 2, 156 (2007)

[2] Kuc, A. et al. Theor. Chem. Acc. 120, 543 (2008)

[3] Kuc, A. et al. Phys. Rev. B: Condens. Matter 74, (2006)

[4] Terrones H. et al., Phys. Rev. Lett. 84, 1716 (2000)

[5] Ramezani H. et al., J. Comput’l & Theror’l Nanoscience 4, 96 (2007)

Strengths and limitations of theoretical approaches used to study carbon nanostructures

Computers have become significantly more powerful since the 1940's. The raise of the computational power has made it possible to use numerical simulations to describe different physical phenomena from theoretical basis. Nowadays computational physics has become an important branch of science, and also carbon nanomaterials have been widely studied from theoretical basis.

Computer simulations have some aspects that are superior to experiments. With computational approach one always knows exactly the composition of the sample, and for example examining the properties of perfect carbon nanotubes is simple. In experiments there is always a possibility that some impurities or defects have found their way in to the sample. Also, making slight modifications to the sample (length of the tube) to see how it affects the results is straightforward with computers. In contrast, producing a specific impurity to the nanotube of certain length and type in experiment is (at least practically) impossible.

Collecting data from simulations is easy and it is possible to investigate properties that are out of reach in experiments. For example the electron density near the atoms of a fullerene inside a carbon nanotube may help to understand why the nanoparticles behave as they do. Therefore simulations can help to explain what is going on in the experiment. Of course it is also very important to look at the properties that can be measured in experiments in order to make sure that the theoretical results agree with the experimental ones.

The most sophisticated and used tool in studying electronic structures from theoretical basis is density functional theory (DFT) [1,2]. It gives very good results with a large variety of systems. Unfortunately it is also computationally heavy method. Therefore studies of nanomaterials with DFT are usually limited to small nanoparticles or bulk calculations. The size limitation causes problems when one wants to imitate an experiment in computer, because in experiments e.g. carbon nanotubes are usually longer than what is reasonable to simulate. Therefore one is often required to build a small system that describes the real system as well as possible with as few atoms as possible.

Fortunately carbon as a material is very convenient for simulations. The electron wave functions of a carbon-only (or hydrocarbon) materials can be very accurately described by using atom-centered basis set consisting of only s- and p-type atomic orbitals. Compared to more sophisticated methods (e.g. DFT with plane wave implementation), this allows one to run simulations of much larger systems and/or longer time periods (the speed compared to DFT may easily be four orders of magnitude faster). These kinds of methods are often called tight-binding approximations and there is a huge number of different kinds of implementations, which tells that the approximation works well in many situations.

Usually these kinds of methods require parametrization of different element pairs. Each parametrization must be tested to make sure that they produce physically sane interaction between elements. The problem with parametrizations is that they are usually tested against some reference systems. In principle there is no guarantee that the parametrization gives reasonable results for systems other than in the test set. However, this is a problem in DFT also; pseudopotentials and certain hybrid functionals contain parameters that are fitted to empirical data to give good results for different test sets.

Even with these kinds of fast, more approximative methods studying time evolution of large systems is still time consuming, and studying for example how a certain reaction happens is nothing but simple. As tempting it would be to start with some configuration of atoms and see what happens as time goes by, one is often limited to study total energies of different configurations at some reaction path and conclude whether the reaction might take place by following that path or not. Of course there are infinite number of reaction paths and one may miss the most important one.

Even with state-of-the-art methods there is always some uncertainty in how much you can trust your results. With DFT, being a ground state theory, all the properties connected to e.g. electron excitations are more or less arbitrary. With further approximations (i.e. a simpler theory) it becomes more and more unclear which properties should come out right. For each system one should think about what is the best tool to describe it. From computational point of view the simplest is usually the fastest, but it may not cover all the crucial physical phenomena required to describe the system well.

[1] P. Hohenberg and W. Kohn, Inhomogenous Electron Gas, Phys. Rev. 1964 volume 136, number 3B

[2] W. Kohn and L. J. Sham, Self-consistent equations including Exchange and correlation effects, Physical Review 1965, volume 140, number 4A

Nature and effect of defects in carbon nanostructures

Currently, many laboratories of the world are engaged with the synthesis of carbon nanostructures. Most recent advances in nanoscience and nanotechnology were made through the fabrication and characterization of novel carbon-based nanostructures such as the fullerenes, carbon nanotubes, and graphenes. It is an indisputable fact that carbon has unique chemical properties among all elements of the periodic table, being essential to construct those nanomachines that existed in the natural world before man’s appearance on earth and work inside the cells of all living organisms [1].

Fullerenes and carbon nanotubes can be formed by rolling up a graphene sheet around a sphere and a cylinder, respectively. Since this discovery, the carbon nanostructures attracted a lot of attention of both experimentalists and theorists because of their unique transport and mechanical properties as a quasi-one-dimensional material with a nanometer-scale dimension.Graphene shows extraordinary electronic transport properties. A graphene sensor was able to detect the absorption of a single gas molecule due to the high sensitivity of its electrical resistance to local changes in charge carrier concentration [1].

The interest of scientists to this new form of carbon is being increased. In this regard, it is very interesting to perform a brief analysis of defects in carbon nanostructures.

There are two main types of defects. The first one is a vacancy obtained by extracting single atom. There are some known facts about the structure and chemistry of the sidewall defect sites that result from the removal of individual carbon atoms. Several different defects are generated by removing one or two carbon atoms from the side wall of a pristine zigzag nanotube. When one carbon atom is removed from the nanotube side wall, three carbons with dangling bonds are formed. A new bond can form between two of these, generating a pentagon.

The second type of defect does not have missing atoms, but has a failure in the atomic arrangement (topological defect), causing two pairs of pentagons among the honeycomb network of hexagons. The latter defect is called a Stone-Wales defect, named after the two scientists who proposed it. This defect attaches to the tube convex and concave surfaces. The tubes are curved and swirl. This Stone-Wales transformation is thought to play an important role during the growth of carbon nanostructures. Once formed, the pentagon/heptagons could move along the structure, creating either dislocation centers in regions of positive (pentagons) or negative (heptagons) Gaussian curvature, which ultimately lead to the closing of the nanostructure. The high activation energy barrier of several electron volts for the bond rotation in nanotubes would make the density of Stone-Wales defects small in thermodynamic equilibrium. Still, these defects should occur as metastable structures at room temperature, and can be frozen in the nanotube body during the nonequilibrium growth process. Note that other energetically comparable, but much more visible defects, including isolated pentagons and heptagons ͑are often seen in transmission electron microscopy images, since these defects induce bending and reshaping of the entire nanotube structure at a relative small cost of elastic strain energy [2].

Stone-Wales defects can be identified by the induction of a defect-localized vibration by photo-excitation [2]. This technique can also be used to monitor the formation of SW defects during nanotube growth [2]. Stone-Wales defects can not be removed.

It was well known that nanotubes are still stable against thermal excitation, even in the presence of these defects. Nanotubes are not so stable against illumination. (This fact may be obtained as a result of a molecular dynamics simulation. However, using this simulation it is not easy to investigate structural changes in materials induced by illumination). However, nanotubes can repair of defects after illumination very fast.

Internal defects and irradiation-induced defects in carbon nanotubes influence on mechanical as well as electronic properties of carbon nanotubes. Regardless of this fact, defects in nanotubes can be useful, for example, for nanotube band engineering, improving mechanical properties of macroscopic nanotube-based materials and nanotube functionalization. Carbon nanotubes and fullerenes have a lot of potential applications in nanotechnology. Defects also influence the transport properties of the nanotubes. Topological defects may occur in the as-grown nanotubes, or they can be generated by several methods like chemical treatment or irradiation with charged particles. Recent experiments show that both electron and heavy ion irradiation can modify the structure and dimensions of carbon nanotubes [3].

[1] E. W. S. Caetano, V. N. Freire, S. G. dos Santos, E. L. Albuquerque, D. S. Galvao, and F. Sato, Defects in Graphene-Based Twisted Nanoribbons: Structural, Electronic, and Optical Properties, Langmuir, 2009, 25 (8), pp 4751–4759.

[2] Yoshiyuki Miyamoto, Angel Rubio, Savas Berber, Mina Yoon, and David Tomanek. Spectroscopic characterization of Stone-Wales defects in nanotubes. PHYSICAL REVIEW B 69, 121413 (R) 2004.

[3] Z. Osváth, G. Vértesy, L. Tapasztó, F. Wéber, Z. E. Horváth, J. Gyulai, and L. P. Biró. Atomically resolved STM images of carbon nanotube defects produced by Ar+ irradiation. PHYSICAL REVIEW B 72, 045429 ͑2005.

Carbon nanostructures beyond fullerenes, nanotubes and graphene: From nanobuds to foams to schwarzite to diamondoids

In this work I try to review different forms of carbon, mainly from chemist's point of view. Main forms are linear carbons – polyyne and polycumulene, glassy carbon, graphyte compounds – such as alkali metals graphitides, graphene oxide, graphite salts. Glassy carbon was discovered accidentally, in 1960s [1]. It is obtained by temperature treatment of polymers, not allowing graphitization [2]. X-ray diffraction analysis shows presence of extremely distorted graphite-like structure. Very small parts of graphite layers are placed chaotically. Crystallites have size like 50x15A, compared with hundreds of angstroms, typical for graphite. Inter-layers distance is 3.5 A versus 3.35 A for graphyte. Such properties as density, porosity, strength shows that sp³ - hybridized atoms are present.
Carbon foams are rather similar to glassy carbon in terms of structure, but demonstrate very low density and they are insulators. Carbon nanofoam was obtained first by Rode in 1999 [3] by laser ablation of carbon in argon. Such environment leads to high percent of sp3 bonds formed. Nanofoam consists of warped graphite-like negative-curved sheets. Nanofoam has 300-400 g/m² surface. sp²/sp³ bonds ratio was studied by EELS method. sp² fraction is near 0.9, which is much lower comparing with glassy carbon.
Linear carbons are somewhat like limiting case of nanotube consists of only one row of atoms. Obtaining of third carbon allotrope was a great challenge for chemistry, because 3D form – diamond and 2D – graphite was known very well. Organic chemistry says that two variants of linear carbon are possible – polycumulene with only double bonds and polyyne with alternating single and triple bonds. They commonly called carbyne. Main way of synthesis is gradual oxidation of C₂H₂ resulting a polyyne. Polycumulene was obtained through polymeric acetylene-allene glycol with former reducing. These structures were confirmed by ozonation analysis and IR spectroscopy. Linear carbons forms hexagonal lattices with parallel threads of carbon. Such structures are studied as object for developing organic conductors.
Next class of carbon forms which I want to review is graphite intercalation compounds. We cant say that this is carbon in strict sense, but they keep sheet structure of graphite. Main classes of graphite intercalation compounds are metal graphitides, such as potassium graphitide KC₈, graphite salts, such as graphite sulfate and covalent compounds such as graphite oxide and graphite acid.
Main feature of such compounds is layered structure. Intercalant atoms or molecules create their own layers between graphite layers. Different amount of intercalate can go into graphite. Limit case is stage 1 intercalate. For potassium, it is KC₈, every other layer is potassium. Graphite layers are bounded as AAAA. Potassium keeps reactivity and readily oxidizes by air and so on.
Graphite sulfate can be obtained by direct syntesis or electrochemically. H₂SO₄ molecules and HSO₄^- ions are intercolated. C₂₄HSO₄x2.5H₂SO₄ for stage 1 and C₄₈HSO₄x2.5H₂SO₄ are known. These compounds are not stable on aur and absorb water with decomposing easily.
Last class of compounds which I want to review is graphene oxide. It is also called graphite oxide, graphitic oxide or graphitic acid. Graphene oxide layers are about 1.1a0.2 nm thick. Scanning tunnelling microscopy shows the presence of local regions where oxygen atoms are arranged in a rectangular pattern with lattice constant 0.27 nm x 0.41 nm The edges of each layer are terminated with carboxyl and carbonyl groups. X-ray photoelectron spectroscopy shows the presence of carbon atoms in the non-oxygenated ring context (284.8 eV), in C-O (286.2 eV), in C=O (287.8 eV) and in O-C=O (289.0 eV). [7] Such compounds are used for graphene synthesis through reduction. Other nano applications are also possible.

References

[1] J. C. Lewis, B. Redfern, and F. C. Cowlard, Solid-State Electronics 6 (1963) 251.

[2] Cowlard, F. C.; Lewis, J. C. (1967). "Vitreous carbon — A new form of carbon". Journal of Materials Science 2 (6): 507, 512.

[3] A.V. Rode, S.T. Hyde, E.G. Gamaly, R.G. Elliman, D.R.. McKenzie, S. Bulcock, Appl. Phys. A 69 (1999) S755.

[4] A. M. Sladkov, V. I. Kasatochkin, Yu. P. Kudryavtsev and V. V. Korshak. Russian Chemical Bulletin, Volume 17, Number 12 / December, 1968

[5] M.S. Dresselhaus, G. Dresselhaus. Intercalation compounds of graphite. Advances in Physics, 2002, Vol. 51, No. 1, 1-186

[6] D. D. L. Chung Graphite review. Journal of Materials Science, Volume 37, Number 8 / April, 2002

[7] D. Pandey and others (2008), Scanning probe microscopy study of exfoliated oxidized graphene sheets. Surface Science, volume 602 issue 9, page 1607-1613

History, controversies and progress in hydrogen storage in carbon nanostructures

Scientists have been interest in using hydrogen as a fuel, but the problem had been in how to store up hydrogen safely and effectively [1]. Hydrogen has been tried to store up in chemical storages, as a compressed hydrogen gas, as a liquid hydrogen, and by gas-on-solid physical adsorption [2], but none of those have been commercially profitable. Today nanoscientists think that answer to this problem might be in nanotubes and in other nanostructures.

One of the first reports about hydrogen adsorption on high-surface-area carbon was reported by Kidnay and Hiza in 1967. They found out that there is at least three ways to amount how much gas is adsorbed to material surface: excess amount, total amount and plotting amount of hydrogen against pressure. Excess amount means 'the excess material present in the pores over that which it would be present under the normal density at the equilibrium pressure' [1]. In total amount all the matter that is influenced by adsorption forces is calculated. It is also possible to just plot the amount of hydrogen contained in the adsorbent-filled container as a function of pressure. These three ways are still used to report the amount of material adsorbed to adsorbent. Kidnay and Hiza reported their maximum excess amount in their experiment to be 20.2-g H2/kg carbon at 25 atm and 76 K, corresponding to a gravimetric storage density of ~ 2.0 wt%, but this profitable enough to store hydrogen. Storage density was too low and keeping such a low temperature would be a problem in daily use.

In 1980 Carpetis and Peschka suggested that hydrogen can be stored inexpensively into activated carbon materials by adsorption in very low temperatures. In their experiment they succeeded to maximize hydrogen adsorption to ~5.2wt% at 41.5 atm and 65 K. But the temperature was too low to store hydrogen commercially profitable this way.

In the late 1980's and early 1990's research group of J.A. Schwarz focused on fundamental aspects of hydrogen adsorption on carbon nanomaterials. Their goal was that operating temperature of the hydrogen adsorption could be increased. To achieve their goal they investigated effects of surface acidity and metal modifications. In their experiment they were able to maximize hydrogen concentration only to 4.8 wt%, at a temperature of 87K and a pressure of 59 atm. The group also carried out detailed thermodynamic analyses and determined important parameters as the isosteric heat of adsorption.

In 1999 Orimo et al. tried to increase the amount of hydrogen storage into carbon sorbent by mechanically ball milling graphite under a hydrogen atmosphere. Orimo et al. were able to get hydrogen concentration to as high as 7.4 wt% after 80 hours of milling. This was the first time when D.O.E.s (United States Department of Energy) target values (6.5 wt% and 62-kg H2/m3) were reached. Problem in this method was that reversibility of charging and discharging was too unlikely.

Recent studies have shown that carbon nanofibers can store hydrogen very effectively. Amount of hydrogen can be as high as 15 wt% in carbon nanofibers [2] which is over double of amount what D.O.E. has set as the target value. Carbon nanofiber technique is based on that hydrogen has a kinetic diameter of 0.289 nm which is smaller than the space between two graphite sheets in carbon nanofiber (~0.335-0.342 nm). When carbon nanofibers are exposed to hydrogen under pressure of 120-130 atm at room temperature hydrogen starts to slide between carbon layers. If the pressure is lowered hydrogen starts to slide off between graphite sheets and form hydrogen gas. So the hydrogen tank can be easily and effectively charged and discharged without using very low temperatures. Problem in this technique is that it needs a very high pressure which can be problematic in daily life.

If carbon nanostructures will be main storage of hydrogen in the future it still has to be polished. Hydrogen adsorption capacity must be maximized at room temperature and under moderate pressures. These tanks must be able to charge and discharge rapidly and completely at near ambient conditions. It also will be necessary to scale up the synthetic and purification techniques to produce ideal hydrogen adsorption materials that can be done in industrial-scale.

[1] Hydrogen storage using carbon adsorbents: past, present and future, A.C. Dillion, M.J. Heben, National Renewable Laboratory, Colorado, USA, 2000 http://www.springerlink.com/content/0hlb80b86ra3rbk4/fulltext.pdf

[2] Carbon nanofibers as a Hydrogen Storage Medium for Fuel Cell Applications in the Transportation Sector, M.A. de la Casa-Lillo, F. Lamari-Darkrim, D. Cazorla-Amoros, A. Linares-Solano, 2002 http://www.sjsu.edu/faculty/selvaduray/page/papers/mate115/robertzeches.pdf

Chemical functionalization of fullerenes and nanotubes, and their applications

Fullerenes and carbon nanotubes have many interesting physical properties. Their mechanical, electrical and structural characteristics are exceptional. Nevertheless there are problems to be solved to unleash their full potential for example in biological applications. One issue is solubility. Carbon nanotubes as prepared are insoluble. Fullerenes are only slightly better and the solvents are not very friendly. With functionalization it is possible to find new exciting application not possible with pristine materials.

The conditions for functionalization are often quite harsh, requiring quite high temperatures, long reaction times, high pressure and using very reactive chemicals such as concentrated sulphuric acid or nitrogen acid.

Even though fullerenes were discovered earlier they fall clearly behind carbon nanotubes in number of applications. One of the most studied area is medical applications of fullerenes. Fullerene derivatives have antiviral activity, they have photodynamic activity, antioxidant activity, they can be used in drug and gene delivery and there are also diagnostic applications (1).

Since C₆₀ is formed from pentagons and hexagons not all double bonds are similar. There are bonds connecting pentagons (6,6) and bonds connecting pentagons to hexagons (5,6). As these bonds have different properties different methods can be used for functionalization. (6,6) bonds can for example be used in Diels-Alder reaction to add cyclic groups (2)

There are three distinct ways to modify carbon nanotubes. Covalently attaching functional groups onto pi-conjugated skeleton of the tube, non-covalent adsorption and endohedral filling of the tube.(3) The following is a examples of few possible ways to functionalize carbon nanotubes and their applications.

CNTs can be hydrogenated via dissolved metal reduction with lithium and methanol in liquid ammonia. With thermogravimetric and mass spectroscopic analysis it was determined that CNT's have stoichiometry of C₁₁H. (4)

As an example of non-covalent adsorption is reversible wrapping of SWCNT's with polymer, polyvinyl pyrrolidone. First SWCNT's were dispersed in water with sodium dodecyl sulfate and sonication. Then polymer was added and after 12h incubation at 50 C the resulting mixture was purified and as a result stable solution of PVP wrapped SWCNT's was produced.

Some possible wrapping arrangements of PVP

Polymer composites are also an example of non-covalent adsorption. Epoxy composites are maybe the most widely studied composite type. One of the first carbon nanotube application available for consumer markets was epoxy composite called Hybtonite.

[1] Bonn et al. Int J Nanomedicine. 2007 December; 2(4): 639, 649

[2] Eguchi et al. Tetrahedron Volume 52, Issue 14, 1 April 1996, Pages 4983-4994

[3]Prato et al. Chem. Rev., 2006, 106 (3), pp 1105, 1136

[4]Pekker et al. J. Phys. Chem. B, 2001, 105 (33), pp 7938–7943

[5]Smalley et al. Chemical Physics Letters Volume 342, Issues 3-4, 13 July 2001, Pages 265-271

Progress in the Synthesis and Formation of Graphene

Graphene is a sheet of carbon atoms in the form of two dimensional hexagonal lattice. Materials such as graphite and carbon nanotubes are composed of graphene sheets. It was presumed that graphene doesn't exist in the free state until 2004 when Novoselov et al. reported preparation of single-layer graphene by applying Scotch tape on a piece of graphite [1]. The produced graphene sheets proved to be of high quality and presented some remarkable properties such as extremely high charge-carrier mobilities and mechanical stiffness, which distinguishes it from monoatomic metallic films.

The Scotch tape method (or mechanical exfoliation) produces micron-size flakes suitable for laboratory research but the scaling of the method for larger areas doesn't seem conceivable. Since the discovery of free-standing graphene more methods have been developed to manufacture graphene and they fall into three categories: chemical vapour deposition (CVD) on metal surfaces [2], epitaxial growth on insulator surfaces (such as SiC) in ultrahigh vacuum [3] and the creation of colloidal suspensions [4].

In the CVD method a thin layer of nickel on a substrate is exposed to a carbonaceous gas in a high temperature (1000ºC). Carbon atoms are formed on the surface and they migrate below the surface to interstitial lattice sites. Then the nickel is cooled down and the carbon atoms precipitate out of the nickel layer and form graphene on the surface. Finally the graphene can be removed from the nickel surface by gentle chemical etching and transferred onto appropriate substrates. The CVD method seems to be the most promising way of synthesizing graphenes of large area and high quality. Three separate groups have reported fabrication of single- and few-layer graphene with areas of square centimetres manufactured with a CVD technique, and transferred to other substrates [2, 5, 6]. Next step in the development of the CVD method is to replace the polycrystalline substrates used by the three groups with single-crystal nickel substrates. There is at least one serious obstacle for the method which is the creation of ripple structures due to the large difference in the thermal expansion coefficients of nickel and graphite. The ripples have to be delt with in order to obtain the planar graphene film topology required for micro-patterning of electronic devices.

Epitaxial growth of graphene is done by fabrication of a SiC crystal from which the silicon atoms at the surface are evaporated away by heating. The carbon atoms reconstruct to form few-layer graphene. The layers are stacked rotationally over each other, which preserves the Dirac properties of single-layer graphene[7]. The process is done in ultrahigh vacuum (UHV) in order to avoid oxidation. This method provides graphene suitable for electronics but the requirement for UHV conditions makes it expensive.

Production of graphenes from colloidal suspensions made from graphite, derivatives of graphite and graphite intercalation compounds is scalable and well-suited to chemical functionalization. The methods using colloidal suspension afford the possibility of low cost and high-volume production of graphene, but of a lesser quality than the aforementioned methods.

One route to graphene sheets is via redox reactions [8]. First graphite is oxidized in the presence of some strong acids and oxidants. Then the graphite oxide is suspended in water and intercalation of water molecules between graphene oxide sheets occurs. Then a homogeneous colloidal suspension of graphene oxide is achieved by simple sonication. Finally the aqueous graphene oxide suspension is reduced and dried to produce an electrically conductive black powder. However the oxygen can't be removed completely and thus pristine graphene is not obtained.

Methods have been developed that avoid the oxygen impurities. One uses intercalants that evaporate explosively when heated thus creating thin graphite flakes that can be further separated with proper intercalants and sonication [9]. The other method relies on solvents for which the solvent-graphene interfacial interaction energy matches that of graphene-graphene [10]. These both methods produce graphenes of higher quality than other chemical routes but they still fall short from yielding pristine graphene.

[1] Novoselov et al., Science 306, 666 (2004).

[2] Kim et al., Nature 457, 706 (2009).

[3] Berger et al., Science 312, 1191 (2006).

[4] Park & Ruoff, Nature Nanotech. 4, 217 (2009).

[5] Reina et al., Nano Lett. 9, 30 (2009).

[6] Cao et al., Preprint at
[7] Xuebin Li, Epitaxial graphene films on SiC: growth, characterization, and devices (Georgia Institute of Technology, 2008).

[8] Stankovich et al., Carbon 45, 1558 (2007).

[9] Li et al., Nature Nanotech. 3, 538 (2008).

[10] Hernandez et al., Nature Nanotech. 3, 563 (2008).

Chirality identification in single walled carbon nanotubes

Introduction

Carbon nanotubes (CNT), especially single walled (SWNT), can be treated as a folded sheet of graphene. The way that the graphene sheet is folded determines the properties of the tube and the structure of the nanotube is described by an integer pair (n,m),  from which also the chiral vector and the diameter of a SWNT can be derived. Because the synthesis of the CNTs always produce a distribution of different chiralities instead of one single chirality, it is important to be able to determine the actual chirality of the tube in the sample. The methods used for the determination of the chirality of CNTs are for example resonant Raman spectroscopy, electron diffraction and high resolution transmission electron microscopy (HRTEM).

Raman spectroscopy has been widely used for characterizing SWNTs because it is one of the most sensitive and informative spectroscopic methods to analyze this kind of nanostructures.[1,2] In particular, surface enhanced Raman spectra (SERS) of CNTs [3] suggested that it could be possible to obtain a Raman spectrum from an individual SWNT. In 2001 Jorio et al. presented an observation of Raman spectrum from a single nanotube.[4] This made it possible to study the dependence on diameter and chirality of each feature of Raman spectra of SWNTs.

In order to analyze the resonance Raman spectra it is necessary to compare the experimental data with prediction or to a plot of the resonance transition energies as a function of tube diameter for all SWNTs.[5] This so called Kataura plot shows that the transition energies Eii of each band are approximately inversely proportional to tube diameter dt.[6] In determining the possible resonant tubes it is usually assumed that the SWNT should have Eii within a resonant window of the energy of used laser El ' 0.10 eV.[7-9] The diameter of the CNT is obtained from RBM position by using equations presented in literature, for example in reference [7].

An electron diffraction pattern from a SWCNT has two sets of principal reflections, appearing as two twisted hexagons. [10] One originates from the top layer and the other from the bottom layer as the electron beam passes through the tube. Due the periodicity in axial direction, the scattering intensities on a set of lines. Though the scattering intensities are observable on all layer lines, the principal reflections of {1 0 0}* type bear the strongest intensities. From these reflections rise to three layer lines above and below the equatorial line, respectively. [10]  The scattering intensity distribution of the lines can be calculated precisely. [11] The positions of the first two (or three in some cases) peaks in the intensity distribution are used to determine the orders of Bessel functions which directly give the chiral indices of the measured tube. [10]

Conventional high-resolution transmission electron microscopy (HRTEM) can produce detailed images of the local SWNT structure.[12] This method has been extended to determine the chirality of a SWNT by a combination of the real space phase restoration and its Fourier transformation. [12,13] However, long time focusing of electron beam on specimen would induce distortion of SWNTs and cause unexpected experimental errors. [14] Fourier transform operation is performed to the intact HRTEM image to obtain its optical diffraction pattern and after this inverse Fourier transform is performed to obtain atomic-resolved lattice image by masking stigmatic diffraction lines related to the tube chirality. Eventually through simulations and comparison to other experimental data, the chiral angle and the diameter of CNT are obtained. [14]

[1] Dresselhaus, M. S.; Eklund, P. C. Adv. Phys. 2000, 49, 705.

[2] Jorio, A.; Pimenta, M. A.; Souza Filho, A. G.; Saito, R.; Dresselhaus, G.; Dresselhaus, M. S. New J. Phys. 2003, 5, 139.

[3] Kneipp, K.; Kneipp, H.; Corio, P.; Brown, S. D. M.; Shafer, K.; Motz, J.; Perelman, L. T.; Hanlon, E. B.; Marucci, A.; Dresselhaus, G.; Dresselhaus, M. S. Phys. Rev. Lett. 2000, 84, 3470

[4] Jorio, A.; Saito, R.; Hafner, J. H.; Lieber, C. M.; Hunter, M.; McClure, T.; Dresselhaus, G.; Dresselhaus, M. S. Phys. Rev. Lett. 2001, 86, 1118.

[5] Dresselhaus, M. S.; Dresselhaus, G.; Jorio, A. J. Phys. Chem. C, 2007, 111, 17893.

[6] Kataura, H.; Kumazawa, Y.; Maniwa, Y.; Umezu, I.; Suzuki, S.; Ohtsuka Y.; Achiba, Y. Synth. Met. 1999, 103, 2555.

[7] Araujo, P. T.; Doorn, S. K.; Kilina, S.; Tretiak, S.; Einarsson, E.; Maruyama, S.; Chacham, H.; Pimenta, M. A.; Jorio, A. Phys. Rev. Lett. 2007, 98, 067401.

[8] Maultzsch, J.; Reisch, S.; Hennrich, F.; Thomsen, C., Phys. Rev. B 2005, 72, 205438.

[9] Jorio, A.; Saito, R.; Hafner, J. H.; Lieber, C. M.; Hunter, M.; McClure, T.; Dresselhaus, G.; Dresselhaus, M. S. Phys. Rev. Lett. 2001, 86, 1118.

[10] Liu, Z.; Qin, L.-C.; Chemical Physics Letters 2005, 408, 75'79

[11] Qin, L.-C. J. Mater. Res. 1994, 9, 2450.

[12] Meyer, R. R.; Friedrichs, S.; Kirkland, A. I.; Sloan, J., Hutchinson, J. L.; Green, M. L. H. J. of Microscopy 2003, 212 Pt 2, 152'157

[13] Friedrichs, S.; Sloan, J.; Green, M.L.H.; Hutchison, J.L.; Meyer, R.R.; Kirkland, A. I. Phys. Rev. B 2001, 64, 045406.

[14] Zhu, H.; Suenaga, K.; Hashimoto, A.; Urita, K.; Iijima, S. Chem. Phys. Lett. 2005, 412, 116-120

Toxicology of carbon nanostructures

Introduction

Fullerenes and especially carbon nanotubes (CNTs) are increasingly leaving the exclusive domain of laboratories and into everyday life. Applications such as displays, fuel cells and composite materials (even cements) have varied levels of contact with human body, and it is reasonable to expect that some of this nanomaterial will be inhaled or ingested, or penetrate through skin and other tissue. Given that industry and private entities don't inherently have the best interest of humankind at their heart, it is the duty of the scientific community to carefully research and evaluate the effects that these new substances have on the metabolism and life-functions, in the medium and long terms.

Use of nanoparticles is, generally speaking, nothing new: gold and other metal nanoparticles were used since ancient times for staining glass, and metallic oxides and silica have been in use in the cosmetic industry, in catalysts etc. for the past few decades [1] [4]. From this to conclude that any nanoparticles made of such oxides are innocuous, would be a mistake, as evidenced by the adverse effects of asbestos [2]. Therefore shape/morphology and size of nanostructures are relevant (also) for their health-related action.

Research challenges

When researching the toxicity of carbon nanostructures, one is facing a few difficulties: in vitro experiments don't necessarily produce results relevant to the functioning of a complex organism and can lead to both over- and underestimates. In vivo experiments, on the other hand, are expensive, especially when looking at long-term effects [1]. Still, in vitro experiments are necessary to understand the mechanisms of toxicity of nanomaterials [3]. Localization, in tissues and organs, of absorbed carbon nanomaterials is difficult, while radiolabeling or fluorescently tagging the nanostructures may alter their properties [3]. One possible solution for radiolabeling was found in 1994, by using ¹⁴C [9].

Another issue is finding proper control samples to test against carbon nanostructures [3]. While carbon black has been used for this purpose, its reactivity (e. g. with markers) may skew measurements.

Light excitation of nanoparticles is of fundamental importance and introduces another variable for the researcher to consider. The excited nanostructures may react with substances present in the ambient, causing the attachment of various functional groups; this presents the need to test the toxicity and bio-action of disparate classes of fullerene or CNT-based compounds. For instance, C₆₀(OH)₂₄ causes increased growth of B subtilis bacteria compared to non-hydroxilated C₆₀ or conrol samples [1]. Furthermore, it is likely that excited fullerenes or CNTs will behave differently in a living organism, than those at the ground state, so the researcher should bear this in mind in some cases. This further complicates the research methodology.

It is not surprising that scientists are encountering such challenges at this stage, as appreciable quantities of fullerenes and CNTs have been produced only recently, and the amount of studies is (as shown in the next section) undergoing exponential growth in the last couple of years.

History of research

The few first published papers on carbon nanomaterials toxicity appear in 1992-1993 [5] [6], and deal with C₆₀ fullerenes. Tokuyama et al. [6] did a pioneering work not only because they performed one of the first studies on toxicity of carbon nanostructures, but also because they researched the photo-activation of fullerenes with regards to bio-activity. The article reports on the study of cytotoxicity of C₆₀ carboxylic acid in vitro, on HeLa S3 cells. A marked cytotoxic activity was observed when the sample was irradiated by 6 W fluorescent "white" light, and none otherwise. Similarly, the triethylamine salt of C₆₀ carboxylic acid showed DNA-cleaving activity only when photo-irradiated. The above compounds were chosen as they are much more soluble in water than C₆₀ itself.

Since carbon nanotubes have been "officially discovered" in 1991, research on their toxicology lags almost a decade. One of the first articles to deal with CNT toxicity is by Huczko et al. [7]; the methodology involved is interesting, as the source material is soot from a fullerene arc generator, containing both C₆₀ and CNTs. Both dermatological and pulmonary tests on guinea pigs were performed, and the paper concludes that no skin irritation or allergy was prodced after 72 hours, nor did the soot with high-content of CNTs cause measurable inflammation in the pulmonary system. In the name of historical accuracy it mast be noted that the same authors have published, the year before (1999), a short communication on their ongoing research [8].

As is the case in the early article by Tokuyama et al. [6], research on carbon nanostructure toxicity often times goes hand-in-hand with fullerene and CNT beneficial bio-activity or medical use. In fact, all throughout the short history of the research in the topic of toxicity, the research in benefits and medical uses of carbon nanostructures went, more or less, at the same speed, and a balanced and comparable if not equal, amount of research data has been produced.

Doing a search for "toxicity study of carbon nanotubes" (without quotes) on Elsevier, produces a remarkable result: about 90% of the articles found in this way, have been published in or after 2006, and as of this essay's writing (mid-August 2009), the number of articles published this year almost equals that of the previous two years. This indicates that the interest in this topic is enjoying an explosive growth.

More recent publications

In reference [10], it is reported that weakly water-soluble C₆₀ is much more cytotoxic to in vitro human cell cultures, than C₆₀(OH)₂₄, Na⁺_2-3[C₆₀O_7-9(OH)_12-15]^(2-3)- or C₃ (C₆₀ derivatized with malonic acid), all of which are highly water-soluble derivatives of C₆₀. The authors acknowledge the importance of light activation of fullerenes, and opted to perform the experiments in the dark. C₆₀ was found to be toxic to human dermal fibroblasts at a LC₅₀ value of 20 ppb, which was 3-5 orders of magnitude less than for the derivatives. A very interesting finding was that the level of toxicity is inverse to the level of derivatization of the C₆₀ cage. After further experiments, the article concludes that the likely mechanism of C₆₀ cytotoxicity is peroxidation of the cellular lipid bilayer, forming peroxy-radicals on its alkene termini, causing its hydrophobicity and perforation. The ability of C₆₀ to provide oxygen radicals was also empirically confirmed.

Monteiro-Riviere et al. [3] performed in vitro experiments on human epidermal keratinocytes by exposing them to multi-walled CNTs (MWCNT). A 0.4mg/ml dose after 48h caused 84% of the cells to be penetrated by MWCNTs, indicating a high dermatological hazard from these materials. Besides this, the article has found widely divergent results when using carbon black controls from different sources/vendors, underlying the need to find correct control materials.

Smith et al. [11] introduced single-walled CNTs (SWCNT), with a surfactant and sonication, into the water tanks with rainbow trouts, and tested against freshwater and water with surfactant only. They find that 0.1-0.5 mg/l SWCNTs cause marked increase in ventilation rate, mucus secretion and enlarged mucocytes on the gills, after 10 days of exposure.

In reference [12], a review is made of current (to October 2005) studies in pulmonary toxicity of inhaled CNTs. It is suggested that depostion and toxicity of CNTs (SW and MW CNTs, CNT "ropes" and other aggregates) increases with the decrease of the nanoparticles' aerodynamic diameter. Another conclusion is that MWCNTs and SWCNTs can cause severe inflammatory and fibrotic reactions.

Conclusion

The few articles presented in this essay all point to potential dangers from exposure to carbon nanostructures. At the same time, carbon nanostructures can be also very useful in medical applications, so the research in this field is doubly rewarding. This research is still young and finding solutions to methodological challenges, but as the research effort expands (and it is expanding exponentially), obstacles will be overcome and more useful data generated.

References

[1] Challa S. S. R. Kumar: "Nanomaterials: Toxicity, Health and Environmental Issues", Wiley-VCH 2007

[2] Roberta C. Barbalace. A Brief History of Asbestos Use and Associated Health Risks. EnvironmentalChemistry.com. Oct. 2004. Accessed on-line: 8/9/2009
http://EnvironmentalChemistry.com/yogi/environmental/asbestoshistory2004.html

[3] Nancy A. Monteiro-Riviere and Alfred O. Inman: "Challenges for assessing carbon nanomaterial toxicity to the skin", Carbon [0008-6223] yr:2006 vol:44 iss:6 pg:1070

[4] Maynard, A.D., Michelson, E. 2005. The nanotechnology consumer products inventory. Woodrow Wilson International Center for Scholars.

[5] Wagemann, K., Baselt, J.P.: Fullerenes - analysis and evaluation A discussion paper prepared within the framework of the analysis and evaluation of new innovative approaches to chemical fundamental research (1992)

[6] Hidetoshi Tokuyama, Shigeru Yamago, Eiichi NakamuraTakashi Shiraki and Yukio Sugiura: Photoinduced Biochemical Activity of Fullerene Carboxylic Acid, J. Am. Chem. Soc. 1993, 115, 7918-7919

[7] Andrzej Huczko, Hubert Lange, Ewa Calko, Hanna Grubek-Jaworska, Pawel Droszcz, Toshiaki Sogabe: On Some Aspects of the Bioactivity of Fullerene Nanostructures, Electrochem. Soc. Proceedings, Vol. 2000-11

[8] A. Huczko, H. Lange, E. Calko:,Short Communication: "Fullerenes: Experimental Evidence for a Null Risk of Skin Irritation and Allergy"; Fullerenes, Nanotubes and Carbon Nanostructures, Volume   7, Issue  5  September 1999, pages 935 - 939

[9] Scrivens WA, Tour JM: Synthesis of ¹⁴C-labeled C₆₀, its suspension in water, and its uptake by human keratinocytes; Journal of the American Chemical Society [0002-7863] yr:1994 vol:116 iss:10 pg:4517

[10] Christie M. Sayes et al.: The Differential Cytotoxicity of Water-Soluble Fullerenes; Nano Letters 2004, Vol. 4, No. 10 pp. 1881-1887

[11] Catherine J. Smith, Benjamin J. Shaw, Richard D. Handy: Toxicity of single walled carbon nanotubes to rainbow trout, (Oncorhynchus mykiss): Respiratory toxicity, organ pathologies, and other physiological effects; Aquatic Toxicology 82 (2007) 94'109

[12] Julie Muller, Francois Huaux, Dominique Lison: Respiratory toxicity of carbon nanotubes: How worried should we be?; Carbon 44 (2006) 1048'1056

The fullerene family and fullerene-based materials

Fullerenes are a relatively new class of nanomaterials that represent a third form of carbon (e.g. diamond and graphite) and are spherical in shape – like a soccer ball. The most common fullerene sphere, called a "Buckyball," contains 60 carbon atoms bound by single and double bonds that form a three-dimensional geodesic spheroidal crystal. For these "empty cages", 60 or 70 carbon molecules are arranged in a cage structure and are water insoluble unless derivatized with hydrophillic compounds. The fullerene family has very appealing properties which can be exploited alone or through the addition of atoms within the cage or by the addition of surface chemistry. (1) There are many identified possibilities for using these molecules in developing new drugs or improving upon current drugs. For example, the compounds can be used for specific targeting of cells and locations within the body after intravenous or subcutaneous injection. Likewise, certain fullerenes, such as the Trimetasphere, contain metallic ions which help increase image quality. And, since these metals are captured within the car-bon cage, release into the body is not a pathway for these toxins – the cage carries the metal throughout elimination. Given the plethora of capabilities and options that fullerenes and Trimetaspheres bring to the field of nanomedicine, it is not surprising the effect it is having on medical research and drug delivery science. Chemists can now produce various derivatives of fullerenes. For example, hydrogen atoms, halogen atoms, or organic functional groups can be attached to fullerene molecules. Also, metal ions, noble gas atoms, or small molecules can be trapped in the cage-like structures of fullerene molecules, producing complexes that are known as endohedral fullerenes. If one or more carbon atoms in a fullerene molecule is replaced by metal atoms, the resultant compound is called a fulleride. Some doped fullerenes (doped with potassium or rubidium atoms, for example) are superconductors at relatively high temperatures. The chemistry of fullerenes, developed in these last years, has allowed designing the properties of this family of molecules for specific applications in materials science. One of the main tasks to build up solid state devices based on fullerenes is the synthesis of materials doped with a highly dispersed and homogeneous distribution of fullerenes. Many of the peculiar photophysical properties, such as the reverse saturable absorption used to obtain a solid state optical limiter, are in fact lost in the aggregates of fullerenes. Sol-gel processing allows preparing inorganic oxides and hybrid organic-inorganic mate-rials at low temperatures and presents an interesting alternative to organic polymers to entrap molecules of the fullerene family in a solid matrix. Porous inorganic solids and aerogels are also important classes of materials that can be synthesized via sol-gel and can act as hosts of fullerenes. (2) Discovery of fullerenes and their soluble derivatives brought revolutionary break-throughs in the fields of organic electronics and material science. Parent fullerene C60 is the most advanced organic n-type semiconductor that showed electron mobilities as high as 5-10 cm2 /V s when measured in organic field effect transistors (OFETs). Organic de-rivatives of fullerenes serve as solution processible n-type semiconductors. I summarised the some literature data of the fullerene-based materials. Electronic circuitry. Fullerene C60 and its derivatives are widely used for design of OFETs and more sophisticated electronic devices such as ring oscillators and other functional integrated circuits. There are few companies on the market that develop smart cards, smart tags and some other consumable electronics built entirely from C60-based transistors. Functionalized fullerenes are materials of choice for low cost printable or-ganic electronics. Organic solar cells based on conventional organic materials showed power con-version efficiencies of less than ~1% in 2000. A remarkable breakthrough in the field was achieved when fullerene-based materials were applied. A rapid evolution of organic photovoltaics resulted in power conversions efficiencies of >5% certified for single cells by Konarka Technologies. Calculations revealed that power conversion efficiencies of 10-14% are feasible for organic solar cells based on appropriate photoactive materials. Organic photodiodes and photodetectors. Photodiodes based on composites of fullerene derivatives and conjugated polymers have advantages of exceptionally high sensitivity, fast photoresponse, low or even zero energy consumption and a low cost. Siemens AG demonstrated position-sensitive photodetectors, two-dimensional image scanners and digital cameras based on arrays of organic photodiodes. These develop-ments are currently used in electronic equipment for medicinal diagnostics, in particular, for X-ray imaging. Organic light emitting diodes (OLEDs) comprising fullerene C60 as electron transporting material will also be considered. Successful application of the fullerene-based materials in organic electronics resulted in appearance of a number of commercialized products that prove unambiguously that “fullerenes are not just beautiful, they are useful". (3) References 1. Fullerene Nanomedicines for Medical and Healthcare Applications. Charles Gause. www.hhmglobal.com

2. Brusatin G., Innocenzi P. Journal of Sol-Gel Science and Technology, Volume 22, Number 3, November 2001, pp. 189-204.

3. Fullerene-based materials for organic electronics. Razumov V.F. www.ioffe.ru/IWFAC/2009/abstr/iwfac09. p010.pdf

Unique applications of mechanical toughness of nanotubes

Introduction to material toughness

When talking about material toughness people are often not sure what it actually means. Mathematically toughness of material is the area underneath stress-strain curve Fig 1 [1]. On the other words it is materials´s ability to absorb mechanical (or kinetic) energy up to failure. Mathematical description is shown in Fig 2. [2].

Figure 1

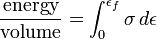
Figure 2

Material strength is closely related to material toughness. On Fig. 1 ultimate tensile strength is the highest point where the line is momentarily flat. So we can see that if we increase steel strength we´ll end up lower total toughness values.

If we take a look to published theoretical and experimental values of nanotubes mechanical properties (for example from Wikipedia) [3] we can see that tensile strength of pure nanotubes is 50-100 times higher than Stainless steel and elognation at break is on almost same level.

When we add the fact that carbon nanotubes have one sixth of Stainless steel density we start to understand how unique the material mechanical properties of nanotubes theoretically are. Combining strength and weight properties of nanotubes to strength/weight ratio the multiplier will be amazing 300-600 related to high or medium carbon stainless steels.

How unique applications we can theoretically make out of such supermaterial can be divided to two ways of thinking. We could use the properties either to make existing constructions ultra light or ultra tough. Ultra light products will help engineers in coming energy consumption challenge e.g building Hydrogen cars, ultraspeed flywheels. Ultra tough properties could lead to constructions which have not been possible before e.g. Space elevator [4]. But because the properties of nanotubes are so good it might mostly be better to distribute the value to both lightness and toughness. In practice it is not likely to be possible to produce materials which consist only of carbon nanotubes. This is the reason why the numbers shown on Table 1 for nanotubes are too optimistic. It is more likely that nanotubes are being used to enhance exisiting materials like concrete, ceramics, plastics and composites. There is already variety of nano-enhanced sport goods like golf shafts, golf balls, tennis rackets available on marketplace and more will come for sure after manufacturing technologies and engineers understanding maturize. On the other hand totally new materials where nanotubes has been used will be and are being developed. For decades material scientist have tried to mimick spider silk to be able to create e.g. lightweight stab or bullet proof fabrics.Thanks to nanotubes It seems that this dream is now closer than ever. Nexia and US Army researchers has reported fabrication of such fiber already 2002 [5]. Another promising result has been reported in 2003 by Nanotech Institute at university of Texas at Dallas [6].

History has showed that it will take still several years or decades before new inventions, in this case the most unique materials, are available in mass production and for normal consumer. I personally hope to see some materials with high wow-facor during my lifeteime. A stab-proof shirt like Frodo weared in J.R.R. Tolkien´s Fellowship of the Rings would be nice.

Nanoweaving of nanotubes

Carbon nanotubes (CNTs) have been known for some time now. Scientist are constantly conjuring up new ideas on how to use them. These range from printable batteries to memory elements in electronics and mixing them in epoxy to form very light composite materials. Because of the great mechanical and electromechanical properties of CNTs they are a very interesting material not only in nanoscale structures but also in macroscale products. One of the ideas involves spinning them into a yarn that can be used in various textiles. The nanotube yarn, in contrast to common carbon fibers, can be bent and even tied into knots without breaking. Therefore it could be used to make fiber supercapacitors [1] or very durable clothing, possibly even bullet-proof vests.

The process of assembling bundles of single-walled carbon nanotubes (SWNTs) into a continuous fiber is actually quite simple [2]. SWNTs produced with the electric-arc technique are dispersed and sonicated in a solution of sodium dodecyl sulfate (SDS), where in optimized concentrations a homogenous dispersion is obtained. Then this solution is injected through a capillary into a stream of a solution containing polyvinylalcohol (PVA). The flow of the PVA solution orients the SWNT-bundles in the direction of the flow and the bundles stick together because the PVA solution does not stabilize against attracting forces between the tubes and bundles like SDS solution does. The result is a continuous ribbon which remains stable. The ribbons can even be washed with pure water to desorb SDS and PVA. The setup used to make nanotube ribbons is shown in Fig. 1.

Nanotube yarn can also be dry spun from multiwalled carbon nanotubes (MWNTs) fabricated using chemical vapor deposition (CVD) -technique [3]. In this method nanotubes are drawn from a MWNT "forest" on a catalyst coated substrate (Fig. 2). The drawn nanotubes are twisted into a yarn very similarly to spinning yarn out of wool or cotton. Hence the geometry of the yarn is similar to that of wool. While typical wool or cotton yarn cross section contains up to about 100 fibers the nanotube yarn contains aout 100,000 MWNTs.

The nanotube yarns are highly flexible and can be tightly knitted and knotted which is a major advantage compared to most polymer fibers, where knotting degrades the strength of the fiber severely [3]. The yarns can also be easily plied like ordinary ropes to make them thicker. SEM images of single-, two ply-, four-ply-, and knitted and knotted MWNT yarns are shown in Fig. 3. The yarns retain their flexibility even when exposed to temperatures of 450°C for long periods or when immersed in liquid nitrogen.

Other properties of nanotube yarns are quite impressive. They can have tensile strengths on the order of 1.8 GPa which matches that of spider silk [1]. The supercapacitors made from coated nanotube fibres can achieve capacitance and energy-storage density of commercial supercapacitors.

The strength, toughness, conductivity, mechanical energy damping capability, flexibility and small diameter (~2% of that of human hair) makes the nanotube yarns a very interesting material for a variety of uses. They could replace metal wires in in electronic textiles and eliminate the rigidity of metal wire -containing textiles. Electronic applications could include distributed sensors, electronic interconnects, electromagnetic shields, antennas and batteries.

[1] Super-tough carbon-nanotube fibers, Alan B. Dalton et al., Nature 423 (2003).

[2] Macroscopic Fibers and Ribbons of Oriented Carbon Nanotubes, Brigitte Vigolo, et al., Science 290 (2000).

[3] Multifunctional Carbon Nanotube Yarns by Downsizing an Ancient Technology, Mei Zhang et al., Science 306 (2004).

Application of nanoparticles in plant biotechnology

Nanotechnology is a collective term for a wide range of relatively novel technologies; the main unifying theme is that it is concerned with matter on the nanometre scale (Greek n˜anos means dwarf). Bionanotechnology or nanobiotechnology is a sub-section of nanotechnology. Bionanotechnology involves the exploitation of biomaterials, devices or methodologies on the nanoscale [1]. In recent years, nanoparticles with sizes typically below 100 nm, have been applied in several fields of bioscience and biomedicine, with an increasing number of commercial applications. Advances have been made in the field of biomedicine, including the development of tools for pathogen bio-detection, tissue engineering and MRI contrast enhancement. Special interest have been focused on those applications developed for targeted delivery of substances and drugs, implying direct movement of nanoparticles to specific organs.

The possibility of targeting the movement of nanoparticles to specific sites of an organism paves the way for the use of nanobiotechnology in the treatment of plant diseases that affect specific parts of a plant. Different procedures have made use of nanoparticles in plants, such as the controlled release of bioactive substances in solid wood and plant transformation through bombardment with gold or tungsten particles coated with plasmidic DNA[2].

One of the most well-known areas of research in plant biotechnology is the application of nanomaterials in the agricultural and food industry, where nanoparticals can be used as new tools for the molecular treatment of diseases, rapid disease detection, enhancing the ability of plants to absorb nutrients etc. Smart sensors and smart delivery systems can help the agricultural industry combat viruses and other crop pathogens. For example, devices could be used to identify plant health issues before these become visible to the farmer. Such devices may be capable of responding to different situations by taking appropriate remedial action. If not, they will alert the farmer to the problem. In this way, smart devices will act as both a preventive and an early warning system. Such devices could be used to deliver chemicals in a controlled and targeted manner in the same way as nanomedicine has implications for drug delivery in humans [3].

The chemical noise arising in ecosystems when using the most of chemicals of plants' protection from pests and pathogens result in unstable state of biocenoses. And this fact in its turn affects adversely steady position of gene pool. Besides, series of chemicals (herbicides, fungicides, germicides, insecticides, synthetic growth-regulating and plant development chemicals) polluting soil, atmosphere and water bodies affect negatively health of people and development of such important branches of productions as plant cultivation, cattle breeding etc [4]. Technologies such as encapsulation and controlled release methods, have revolutionised the use of pesticides and herbicides. Many companies make formulations which contain nanoparticles within the 100-250 nm size range that are able to dissolve in water more effectively than existing ones (thus increasing their activity). Other companies employ suspensions of nanoscale particles (nanoemulsions), which can be either water or oil-based and contain uniform suspensions of pesticidal or herbicidal nanoparticles in the range of 200-400 nm. These can be easily incorporated in various media such as gels, creams, liquids etc, and have multiple applications for preventative measures, treatment or preservation of the harvested product. One of the world’s largest agrochemical corporations, Syngenta, is using nanoemulsions in its pesticide products [3].

In my area of research the most interesting application of nano biotechnology is the process of delivering genes into plant tissue. This process involves two stages. First the gene is inserted into plant cell tissue, and then afterwards chemicals are introduced into the plant to trigger the gene’s function. The two-stage process has been imprecise and the presence of chemicals can be harmful to the plant. Torney et al. made the solution of this problem, using mesoporous Nanoparticles. These Nanoparticles both introduce the gene and activate it at the same time, in a precise and controlled manner and without toxic after effects. Scientists could potentially use this as an aid in imaging analysis of plants which have been activated with the appropriate materials.

Earlier Lin’s research group [5] developed technology with the use of porous, silica nanoparticle system. Spherical in shape, the particles have arrays of independent porous channels. The channels form a honeycomb-like structure that can be filled with chemicals or molecules.

In previous studies, this group successfully demonstrated that the caps can be chemically activated to pop open and release the cargo inside of animal cells. This unique feature provides total control for timing the delivery The team's first attempt to use the porous silica nanoparticle to deliver DNA through the rigid wall of the plant cell was unsuccessful. The technology had worked more readily in animals cells because they don't have walls. The nanoparticles can enter animal cells through a process called endocytosis - the cell swallows or engulfs a molecule that is outside of it. The biologists attempted to mimic that process by removing the wall of the plant cell (called making protoplasts), forcing it to behave like an animal cell and swallow the nanoparticle. It didn't work. They decided instead to modify the surface of the particle with a chemical coating. The coating induces the plants to swallow the particles, effectively ingesting them inside the plant cell walls where the genes could be inserted. Most plant transformation, however, occurs with the use of a gene gun, not through endocytosis. In order to use the gene gun to introduce the nanoparticles to walled plant cells, the chemists made another clever modification on the particle surface. They synthesized even smaller gold particles to cap the nanoparticles. These "golden gates" not only prevented chemical leakage, but also added weight to the nanoparticles, enabling their delivery into the plant cell with the standard gene gun. The biologists successfully used the technology to introduce DNA and chemicals to Arabidopsis, tobacco and corn plants.

Thus, being able to penetrate the cell wall of the plant enables biologists to view the world of plant biology in all of its complex and intricate detail, opening vast new frontiers of discoveries for agriculture and other industries that rely on biotechnology.

[1] Nicole F. Steinmetz and David J. Evans, Utilisation of plant viruses in bionanotechnology, Organic & Biomolecular Chemistry (2007).

[2] Eduardo Corredor, Pilar S Testillano, María-José Coronado, Nanoparticle penetration and transport in living pumpkin plants: in situ subcellular identification, BMC Plant Biology (2009).

[3] Francois Torney, Brian G. Trewyn, Victor S.-Y. Lin, and Kan Wang, Mesoporous Silica Nanoparticles Deliver DNA and Chemicals into Plants, Nature Nanotechnology (2007).

[4] Muzaffar Sharipov, Bio-nanotechnology in preparation of rice seeds and increase of tolerance of rice crop to diseases and unfavorable environmental factors (unpublished).

[5] Supratim Giri, Brian G. Trewyn, Victor S.-Y. Lin, Mesoporous Silica Nanomaterial-based Biotechnological and Biomedical Delivery Systems, Nanomedicine (2007).

Application of carbon nanotubes in biomedicine

Carbon nanotubes are a new class of nanomaterials that have immense potential in the field of biomedicine. The application of CNT in the field of carrier-mediated delivery has become possible after the recent discovery of their capacity to penetrate into the cells. Besides their versatile physicochemical features enable the covalent and noncovalent introduction of several pharmaceutically relevant entities and allow for rational design of novel candidate nanoscale constructs for drug development. CNTs can be functionalized with different functional groups to carry simultaneously several moieties for targeting, imaging, and therapy [1-3]. Functionalized carbon nanotubes (f-CNT) have been demonstrated to deliver proteins, nucleic acids, drugs, antibodies and other therapeutics. So besides the of CNT applications as biosensors, composite materials, molecular electronics and so on, one use of CNTs is as new carrier systems for the delivery of therapeutic molecules and tissue engineering.

This essay focuses on applications of carbon nanotubes in drug delivery and tissue engineering

Drug delivery is a rapidly growing area that is now taking advantage of nanotube technology. This is due to the fact that many potential low molecular weight drugs have bad bioavailability, pure solubility, small half-life and intrinsic toxicity [4]. Systems being used currently for drug delivery include dendrimers, polymers, and liposomes, but carbon nanotubes present the opportunity to work with effective structures that have high drug loading capacities and good cell penetration qualities. These nanotubes function with a larger inner volume to be used as the drug container, large aspect ratios for numerous functionalization attachments, and the ability to be readily taken up by the cell [1]. Because of their tube structure, carbon nanotubes can be made with or without end caps, meaning that without end caps the inside where the drug is held would be more accessible. The drug encapsulation has been shown to enhance water dispersibility, better bioavailability, and reduced toxicity. Encapsulation of molecules also provides a material storage application as well as protection and controlled release of loaded molecules [5]. All of these result in a good drug delivery basis where further research and understanding could improve upon numerous other advancements, like increased water solubility, decreased toxicity, sustained half-life, increased cell penetration and uptake, all of which are currently novel but undeveloped ideas. It should be noticed that the possibility of developing CNT for biomedical applications became a reality after a series of powerful methodologies for their functionalization were described [6]. Different approaches have been proposed to render the nanotubes soluble and compatible with physiological conditions. This is a fundamental issue for their integration into living system environments. A critical parameter to determine biocompatibility is the degree of toxicity of all CNT materials. This is a key issue which is currently under careful and extensive examination from various laboratories. Observations reported by many groups have shown that functionalization remarkably reduces the cytotoxic effects of CNT while increasing their biocompatibility [1]. The evidence gathered so far highlights that the higher the degree of CNT functionalization, the safer is the material, particularly compared to pristine, purified CNT, thus offering the potential exploitation of nanotubes for drug administration. The CNT functionalized covalently with small molecular weight molecules seem to penetrate plasma membranes to a considerable extent via an energy-independent mechanism and cross the membrane in a passive way acting like tiny needles. For example, carbon nanotubes could be functionalized with antibiotics. Amphotericin B is an antimycotic agent used against particularly resistant fungal strains [1]. It is, however, of limited use because it is highly toxic to mammalian cells, likely due to its low solubility in water and its tendency to aggregate and form pores in the cell membrane. The conjugation of amphotericin B to carbon nanotubes could modulate its properties in terms of toxicity and antimycotic efficiency. Another application of CNT is the anti-cancer therapy. For instance, f-CNT could contains a methotrexate molecule together with a fluorescein probe. Methotrexate is a well-known and potent anticancer agent, used also to cure autoimmune diseases [1] However, methotrexate suffers of low bioavailability and toxic side effects. Therefore, an increased bioavailability and a targeted delivery are highly desirable. f-CNT might offer the possibility for improving bioavailability and, in the presence of a targeting unit, to address specifically cancer cells. Besides, single-walled CNT were functionalized with a substituted carborane cage for boron neutron capture therapy. The biodistribution study on different tissues showed that water-soluble carborane nanotubes were concentrated more in tumor cells than in other organs when administered intravenously. These results were preliminary although also promising for future applications of carbon nanotube boron-based agents for effective treatment of cancer [1]. Another class of carbon nanotube-based therapeutic candidates consists of their constructs with synthetic peptide for immune system activation. The decoration of functionalized nanotubes with B and T cell peptide epitopes can generate a multivalent system able to induce a strong immune response [1]. Peptides can be connected to the tubes using the chemoselective approach.

Conclusion

Although applications of nanotechnologies in biomedicine are only in the early stages of development, the possibilities offered by using these nanomaterials for treatment and diagnosis of CNS disorders are outstanding. Various nanomaterials and nanodevices can be used in neural regeneration, neuroprotection, and targeted delivery of drugs and macromolecules across the blood–brain barrier, in tissue engineering and many other. These systems have significant potential for clinical applications. All together, these works lay an important foundation for future studies into improving diagnosis and therapy of human disease.

[1] M. Prato et al., Acc.Chem.Res. 41, 60 (2008).

[2] R.P. Feazell et al., J.Am.Chem.Soc. 129, 8438 (2007).

[3] S.S. Suri et al., J.Occupational Medicine and Toxicology 2, 16 (2007).

[4] J.E. Kipp Int. J. Pharm. 284 109 (2004).

[5] A. Kulamarva Nanotechnology. 20, 25612 (2009).

[6] D. Tasis Chem. Rev. 106, 1105 (2006).

[7] R. Vasita Inter.J. of Nanomedicine. 1, 15 (2006).

Raman spectroscopy of graphene

Introduction

Since the experimental discovery of graphene in 2004 [1], Raman spectroscopy has played an important role in the research of few-layer graphene. Scientists familiar with carbon nanotubes knew that Raman analysis was a powerful tool in chirality determination, which probably led to the rapid adoption of the Raman technique for graphene samples. Initially, Raman spectroscopy was mostly used to determine the number of layers in a graphene sheet, as it's a non-invasive and high-throughput method for sample characterization. Later on, more versatile and insightful experiments have been constructed to better utilize the vast potential of Raman measurements.

Raman spectroscopy is based on the study of inelastic scattering of photons when they interact with elementary excitations of a target material. Such an excitation is typically a phonon, but it can also be a polariton, a plasmon, a coupled plasmon–phonon mode, or a single electron or hole excitation. The amount of energy transferred between the incident photon and the sample corresponds to an energy shift—and thus a frequency shift—in the scattered photon. A plot of the intensity of the scattered light as a function of this energy shift is called a Raman spectrum and can provide information on the lattice dynamics and electronic properties of the sample. This Raman effect was predicted by Adolf Smekal in 1923 but was first observed by Sir Chandrasekhara Raman in 1928, using a filtered beam of sunlight as the photon source and his eye as the detector. Modern setups typically use one or more semiconductor lasers operating in the visible, near-infrared, or near-ultraviolet regime and a charge-coupled device (CCD) for multichannel detection. [2]

The Raman spectrum of high-quality graphene (and graphite) has two characteristic peaks that stand out (Fig. 1), called the G-peak and the 2D-peak (some earlier sources refer to the 2D-peak as the G'-peak). The G-peak corresponds to an optical phonon in the center of the Brillouin zone (Γ) with an energy shift of ~1580 1/cm which is slightly dependent on sheet thickness, chemical and electrostatic doping, and temperature. The origin of the 2D-peak is a bit more complicated, as it involves the creation of an electron–hole-pair near the K point. The excited electron (or the hole) is then scattered twice by zone-boundary phonons with equal energies (~1335 1/cm) but opposite momenta. When the electron–hole-pair recombines, a photon is emitted with an energy that is shifted ~2670 1/cm relative to the incident photon, giving rise to the 2D-peak. Because the wave vector is conserved in every step of the process and the wave vector of light (~10⁵ 1/cm) is much smaller than that of the Brillouin zone boundary (~10⁸ 1/cm for graphene), it follows that no first-order D-peak will emerge from a perfect infinite lattice. However, near the edges of a sheet and in defective graphene, one of the electron (or hole) scattering events can be elastic, resulting in a visible D-peak at ~1335 1/cm. As the D- and 2D-peaks are directly related to the band structure of graphene near the K points, their energy shifts and line shapes depend on the incident photon energy and on the electronic band details. [3,4]

The evolution of graphene's band structure with an increasing number of layers enables one to determine the thickness of a few-layer graphene sheet based on the 2D-peak line shape (Fig. 2). Monolayer graphene has one valence band and one conduction band, and due to their quasi-linear nature near K the possible electron and hole scattering resonances have almost exactly the same energy. Therefore, monolayer graphene has a rather narrow single-component 2D-peak. When the number of layers increases, the electronic bands split, enabling several transitions with slightly different energies. Bilayer and trilayer 2D-peaks can be fitted with 4 and 6 Lorentzian components, respectively, and eventually in bulk graphite the infinitely many components form a continuum band that seemingly consists of two wide Lorentzians [4]. In addition to thickness determination, Raman spectroscopy can be used to extract information on lattice defects and impurities on the sheet, utilizing the existence and intensity of the D-peak. With micro-Raman equipment this type of analysis can be refined to include spatial resolution, allowing the examination of a sheet's homogeneity. The shift of the G-peak has been used to provide information on chemical and electrostatic doping of graphene devices [5,6], as well as to determine that the thermal conductivity of a suspended monolayer is astonishingly high, ~5000 W/mK [7]. Raman spectroscopy also enables the study of stacking order in multilayer graphene samples [8], electron and phonon dispersion measurements by varying the incident photon energy [9], and possibly even chirality determination of graphene nanoribbons [10]. All in all, Raman spectroscopy is probably the single most versatile tool for probing graphene at the moment, with no decline visible on the horizon.

[1] K. S. Novoselov, A. K. Geim, S. V. Morozov, D. Jiang, Y. Zhang, S. V. Dubonos, I. V. Grigorieva and A. A. Firsov, "Electric Field Effect in Atomically Thin Carbon Films", Science 306, 666–669 (2004).

[2] G. Bauer and W. Richter (Eds.), Optical Characterization of Epitaxial Semiconductor Layers (Springer-Verlag, Berlin Heidelberg, 1996).

[3] A. C. Ferrari, "Raman spectroscopy of graphene and graphite: Disorder, electron–phonon coupling, doping and nonadiabatic effects", Solid State Communications 143, 47 (2007).

[4] L. M. Malard, M. A. Pimenta, G. Dresselhaus and M. S. Dresselhaus, "Raman spectroscopy in graphene", Physical Reports 473, 51 (2009).

[5] C. Casiraghi, S. Pisana, K. S. Novoselov, A. K. Geim and A. C. Ferrari, "Raman fingerprint of charged impurities in graphene", Applied Physics Letters 91, 233108 (2007).

[6] J. Yan, Y. Zhang, P. Kim and A. Pinczuk, "Electric Field Effect Tuning of Electron-Phonon Coupling in Graphene", Physical Review Letters 98, 166802 (2007).

[7] A. A. Balandin, S. Ghosh, W. Bao, I. Calizo, D. Teweldebrhan, F. Miao and C. N. Lau, "Superior Thermal Conductivity of Single-Layer Graphene", Nano Letters 8(3), 902 (2008).

[8] L. G. Cançado, K. Takai, T. Enoki, M. Endo, Y. A. Kim, H. Mizusaki, N. L. Speziali, A. Jorio and M. A. Pimenta, "Measuring the degree of stacking order in graphite by Raman spectroscopy", Carbon 46, 272-275 (2008).

[9] D. L. Mafra, G. Samsonidze, L. M. Malard, D. C. Elias, J. C. Brant, F. Plentz, E. S. Alves and M. A. Pimenta, "Determination of LA and TO phonon dispersion relations of graphene near the Dirac point by double resonance Raman scattering", Physical Review B 76, 233407 (2007).

[10] J. Zhou and J. Dong, "Vibrational property and Raman spectrum of carbon nanoribbon", Applied Physics Letters 91, 173108 (2007).

Effect of interlayer coupling and edges on the electronic structure of graphene and graphite

Graphene is a flat layer of carbon atoms arranged in a hexagonal lattice with two carbon atoms per unit cell. It is a monolayer of graphite. Understanding the electronic structure of graphene is the starting point for finding the band structure of graphite. Of the four valence states, three sp2 orbitals form a state with three neighboring carbon atoms, and one p orbital develops into delocalized and states that form the highest occupied valence band and the lowest unoccupied conduction band. The and states of graphene are degenerate at the corner (K point) of the hexagonal Brillouin zone (BZ) (pic.1). Undoped graphene is a semimetal (or zero-gap semiconductor), because although there is a state crossing at Fermi energy, the density of states there is zero and conduction is possible only with thermally excited electrons at finite temperature. Moreover, the particular band structure at the BZ boundary (that is, a linear dispersion) leads to zero effective mass at the point where the valence and conduction band meet together (six points) [1]. Due to this linear dispersion relation at low energies, electrons and holes near these six points, two of which are inequivalent, behave like relativistic particles described by the Dirac equation for spin 1/2 particles (Dirac fermions) [2].

Pic.1 Calculated band structure of graphene [3]

In the structure of graphite, graphene hexagons are stacked in AAA, ABA, and ABC stacking modes. The strong in-plane C-C bonds and weak interlayer interactions are present. The origin of the strong intralayer interaction is the combination of sp2 and bonds. The weak interlayer interactions are due to the overlap of bonds between adjacent graphene layers (see a bonding structure of a single graphene layer on picture 2) [4]. The interlayer orbital interactions are responsible for the conductivity in graphite, since without them there are would be zero-density of states at Fermi level. So to find out the band structure of graphite the possible model (here tight-binging plus dispersion model is considered) should take into account tight-binging description of intra- and interlayer interactions with description of interlayer dispersion.

If the forms of graphite – AAA, ABA and ABC are compared from the tight-binging dispersion model (pic. 3), one can conclude, that in ABA graphite the tight-binding contribution to the calculated interlayer energy is anisotropic in ABA stacking over AAA, whereas the dispersion contribution is more isotropic and does not discriminate between the stacking forms.

The result of the modeling is shown on pic.4. Here the dispersion interaction potential was counted in a form of the Tang-Toennies function:

Pic4. Occupied band structure of ABA graphite.

One can see that the splitting of -band of ABA graphite occurs in reciprocal space. There is no such splitting for AAA graphite [5].

[1] T. Ohta, A. Bostwick, T. Seyller, K. Horn, E. Rotenberg. Controlling the Electronic Structure of Bilayer Graphene. Science 313, 951 (2006).

[2] G.W. Semenoff Condensed-Matter Simulation of a Three-Dimensional Anomaly. Physical Review Letters 53: (1984)

[3] G.W. Hanson. Fundamentals of nanoelectronic. (2008)

[4]W.A. Goddard. Handbook of nanoscience, engineering and technology. (2002)

[5]A. H. R. Palser. Interlayer interactions in graphite and carbon nanotubes. PCCP (1999)

Identifying and modifying the edge structure of graphene

Graphene is a one-atom-thick planar sheet of sp2-bonded carbon atoms that are densely packed in a honeycomb crystal lattice. It can be viewed as an atomic-scale chicken wire made of carbon atoms and their bonds. The name comes from GRAPHITE + -ENE; graphite itself consists of many graphene sheets stacked together.

The carbon-carbon bond length in graphene is approximately 0.142 nm. Graphene is the basic structural element of some carbon allotropes including graphite, carbon nanotubes and fullerenes. It can also be considered as an infinitely large aromatic molecule, the limiting case of the family of flat polycyclic aromatic hydrocarbons called graphenes.

Measurements have shown that graphene has a breaking strength 200 times greater than steel, making it the strongest material ever tested

Graphene has attracted great attention not only because it is the ideal material to study the fundamental properties of 2D nanostructures, but also for its potential applications in future electronic devices. The exceptionally high crystallization and unique electronic properties make graphene a promising candidate for ultrahigh speed nanoelectronics

Graphene nanoribbons (GNR) have been receiving remarkable attention. It was predicted that GNR with certain edge chirality would open the bandgap and show distinguish magnetic, optical and superconductive properties. The bandgap opening of GNR has already been experimentally verified.

All these peculiar properties are strongly dependent on the edge chirality (zigzag or armchair). Current technique to fabricate such well defined GNR is e-beam lithography from graphene sheet, in which the determination of the graphene crystal orientation and edge chirality is highly desired. There are several conventional methods: TEM, XRD, STM and other.

Transmission electron microscopy (TEM) is a microscopy technique whereby a beam of electrons is transmitted through an ultra thin specimen, interacting with the specimen as it passes through. An image is formed from the interaction of the electrons transmitted through the specimen; the image is magnified and focused onto an imaging device, such as a fluorescent screen, on a layer of photographic film, or to be detected by a sensor such as a CCD camera.

TEMs are capable of imaging at a significantly higher resolution than light microscopes, owing to the small de Broglie wavelength of electrons. This enables the instrument to be able to examine fine detail - even as small as a single column of atoms, which is tens of thousands times smaller than the smallest resolvable object in a light microscope. TEM forms a major analysis method in a range of scientific fields, in both physical and biological sciences. TEMs find application in cancer research, virology, materials science as well as pollution and semiconductor research.

Scanning tunneling microscopy (STM) is a powerful technique for viewing surfaces at the atomic level. STM probes the density of states of a material using tunneling current. For STM, good resolution is considered to be 0.1 nm lateral resolution and 0.01 nm depth resolution. The STM can be used not only in ultra high vacuum but also in air and various other liquid or gas ambients, and at temperatures ranging from near zero kelvin to a few hundred degrees Celsius. The STM is based on the concept of quantum tunnelling. When a conducting tip is brought very near to a metallic or semiconducting surface, a bias between the two can allow electrons to tunnel through the vacuum between them. For low voltages, this tunneling current is a function of the local density of states (LDOS) at the Fermi level, Ef, of the sample. Variations in current as the probe passes over the surface are translated into an image. STM can be a challenging technique, as it requires extremely clean surfaces and sharp tips.

X-ray scattering techniques are a family of analytical techniques which reveal information about the crystallographic structure, chemical composition, and physical properties of materials and thin films. These techniques are based on observing the scattered intensity of an X-ray beam hitting a sample as a function of incident and scattered angle, polarization, and wavelength or energy.

But all these methods are destructive, very time consuming or nearly impossible to locate such small regions of interest. The edge scattering would also prevent clear observation in some techniques, such as STM. The increasing interests in graphene demand a fast and non-destructive method to determine the chirality of edges and the crystal orientation of graphene sheet.

Raman spectroscopy is a proper candidate for this method. As one of the most commonly used techniques to characterize carbon related materials, Raman spectroscopy plays a very important role in acquiring information on the physical, chemical and even electronic properties of graphene and graphene based devices. It helps to determine the edge chirality, hence the crystal orientation of graphene using the difference in intensity of the disorder-induced D band on the different chiralities of edges (stronger in armchair edges and weaker in zigzag edges). This provides an easy and nondestructive method to identify the edge chirality of graphene, which would help to speed up the practical applications of graphene nanoelectronic devices, such as GNR.

[1] Y. M. You, Z. H. Ni, T. Yu, Z. X. Shen, "Edge chirality determination of graphene by Raman spectroscopy", http://arxiv.org/pdf/0810.4981

[2] Kyle A. Ritter, Joseph W. Lyding, "The influence of edge structure on the electronic properties of graphene quantum dots and nanoribbons", Nature Materials, 8 (3), (2009)

[3] C. Lee et al. "Measurement of the Elastic Properties and Intrinsic Strength of Monolayer Graphene". Science, 321- 385, (2008).

[4] M.Y. Han, B. Özyilmaz, Y. Zhang, and P. Kim "Energy Band-Gap Engi-neering of Graphene Nanoribbons", Phys. Rev. Lett. 98, 206-805, (2007).

Impact of carbon nanotubes on Pakistan economy

Carbon nano tubes are of great interest for researchers nowadays .The advancement in the carbon nano tubes is going to play a key role in the economy of every country. I would like to discuss about the impact of carbon nano tubes on the Pakistan economy in future. The Pakistan main exports are based on textile products about 68% which play a key role in Pakistan economy. The trend is going in textile these days as the consumers demand more light and good quality material generally with natural cotton fibers, polyester or some other man made product is mixed in order to improve the strength and properties. ' one plus one can become eleven also and need not necessarily be two always,' as a famous phrase. Recently due to the discovery of worlds hardest plastic nano- composite material which is created by reinforcing ordinary plastic with diamonds which are invisible to naked eye, a sheet of layered carbon and tiny carbon cylinders. Strengthening a common polymer, polyvinyl alcohol. With nano-diamond, a new age material called graphene (one atom thick carbon honeycomb sheet) and carbon nano tube has produced this material with extra hardness and stiffness. By using this material with natural cotton the properties can be increased many folds and it can bring a revolution in the textile world. By using this the Pakistani textile can get a leading position in the textile world and the economy will boost exponentially.

The mechanical properties like hardness and stiffness (after moulding) improved by as much as 400 per cent compared to those obtained with single reinforcements,' By exploiting this property the Pakistani sports industry which is the 2nd big export industry can become the best in the world as the Pakistani sports products are well known all over the world. A simple recent example is of hockey stick which is made by using nano carbon tubes in composite which is of great interest these days all over the world. Despite its hardness the material is extremely light-weight. Despite being the world's hardest plastic nano-composite, the reinforcement material constitutes only one per cent (by weight) of the composite. To create a similar material conventionally, 50-60 per cent glass was required as the reinforcing agent and the resultant composite could not be molded. Besides excellent mechanical properties, the reinforced polymer also shows semi-conducting behavior, which could also be exploited. However, researchers are yet to analyze the new material's toughness and ductility, without which the extent of practical application could not be decided.

The other big export products of Pakistan are ceramics products. A ceramic material reinforced with carbon nano tubes has been made by materials scientists. The new material is far tougher than conventional ceramics, conducts electricity and can both conduct heat and act as a thermal barrier, depending on the orientation of the nano tubes. The researchers mixed powdered alumina (aluminum oxide) with 5 to 10 percent carbon nano tubes and a further 5 percent finely milled niobium. Carbon nano tubes are sheets of carbon atoms rolled up into tiny hollow cylinders. With diameters measured in nanometers -- billionths of an inch -- they have unusual structural and conducting properties. The material shows electrical conductivity ten trillion times greater than pure alumina, and seven times that of previous ceramics made with nano tubes. It also has interesting thermal properties, conducting heat in one direction, along the alignment of the nano tubes, but reflecting heat at right angles to the nano tubes, making it an attractive material for thermal barrier coatings.

Carbon nano tubes can also be exploited in defense sector. The composite material made from nano carbon tubes having a great mechanical strength can be used in airoplanes,missiles,bullet proof jackets for army and helmets which will be lighter and more strengths .In short using the carbon nano tubes Pakistani economy can boost exponentially and have a positive impact on inhabitants lives.

Progress in the synthesis of carbon fullerenes and nanotubes

Fullerenes

Fullerenes are the only soluble form of carbon. Some scientists applied them for technological tasks, others for the synthesis of new materials. Fullerenes would arouse more interest, if they were cheaper and more available. Thus, obtaining an effective technique of fullerene synthesis remains an actual task. At present the arc method of carbon transformation from graphite to fullerenes is considered to be more effective [1]. There are several techniques to carry out with arc method. Some research group operates in high pressure condition with various gases. On the other hand, some scientists make it in low pressure with proper gases or even in vacuum. The schematics of different pressure conditions are shown as Fig. 1(a) and 1(b) [2, 3].

(a)

(b)

In addition, the laser vaporization method shown in Fig.2, which had been originally used as a source of clusters and ultrafine particles, was developed for fullerene and CNT production by Smalley’s group [3].

Fig.2 Schematics diagram of the laser-furnace apparatus [3].

In the same way as what happened with fullerene, after carbon nanotubes were discovered in this world, most scientists have been interested in this nanostructure until nowadays due to its miracle properties provided to many of applications not only in physics and chemistry but also in biology even in medical science. However, there are still a few drawbacks of the synthesis technique being solved by scientists such as the high cost, low uniformity and productivity. However, there are at least 3 techniques to fabricate carbon nanotubes namely arc discharge, laser ablation and chemical vapor deposition (CVD). Both arc discharge and laser ablation methods can be simply described by Fig.1 and Fig.2. Differently from both ways, by adding the catalysts in the system, one can get carbon nanotubes as well. This is so-called CVD method yielding the most productive so far. The common instrument is shown in Fig.3 [4].

At present, the world’s longest carbon nanotube arrays carried out by CVD technique have been done by researchers at the University of Cincinnati [5].They are slightly less than 2 centimeters long and each nanotube is 900,000 times longer than its diameter shown in Fig.4.

Fig.4 The longest carbon nanotube arrays in the world [6].

On the other hand, the length of individual carbon nanotube has been produced beyond 2cm long. The reports of the length comparing with various years and places are shown in Fig.5 (a) and (b) [7].

(a)

(b)

[1] G.N. Churilov, Synthesis of fullerenes and other nanomaterials in arc Discharge, Invited Lectures.

[2] http://nobelprize.org/nobel_prizes/chemistry/laureates/1996/illpres/carbon.html

[3] Yoshinori Ando, Xinluo Zhao, Toshiki Sugai, and Mukul Kumar, Growing Carbon Nanotubes, materialstoday October 2004.

[4] http://ipn2.epfl.ch/CHBU/NTproduction1.htm

[5] http://www.uc.edu/News/NR.aspx?ID=5700

[6] http://www.nsf.gov/news/news_images.jsp?cntn_id=108992&org=NSF

[7] P.J. Burke, C. Rutherglen, Z. Yu, Single-Walled Carbon Nanotubes: Applications in High Frequency Electronics, International Journal of High Speed Electronics and Systems Vol. 16, No. 4 (2006) 977-999.

Distinguishing valuable nanostructures from worthless junk: benefits and limitations of experimental techniques

A nanostructure is a device of the order of a nanometer in its smallest dimension. One nanometer is about a few atoms thick, or, a hundred thousand times thinner than human hair. Existing commercial devices, such as the Pentium Processors, contain submicron structures, which are about 100 times larger than nanostructures linearly, and about 10,000 times larger in area. We can put more components that are much faster and more energy efficient in a device of the same size. It is said to be the whole world [1].

The technical difficulty of fabrication increases, making the process less reliable. What's worst, since we also want to put more components in the same device, the probability of getting a fault component in a device increases dramatically. These are experimental and technical challenges.

There are several experimental techniques which can be used to produce nanostructures: Methods for Bulk Synthesis of Carbon, Pulsed Laser Vaporization, High-pressure CO conversion and Chemical Vapor Deposition. All of these methods are commonly used in manufacturing nanotubes, nanofiber, nanoflake and so on.

The arc-evaporation method, which perhaps produces the best quality nanotubes, involves passing a current of about 50 amps between two graphite electrodes in an atmosphere of helium. This causes the graphite to vaporise, some of it condensing on the walls of the reaction vessel and some of it on the cathode. It is the deposit on the cathode which contains the carbon nanotubes. Single-walled nanotubes are produced when Co and Ni or some other metal is added to the anode. Carbon nanotubes can also be made by passing a carbon-containing gas, such as a hydrocarbon, over a catalyst. The catalyst consists of nano-sized particles of metal, usually Fe, Co or Ni. These particles catalyse the breakdown of the gaseous molecules into carbon, and a tube then begins to grow with a metal particle at the tip. It was shown in 1996 that single-walled nanotubes can also be produced catalytically. The perfection of carbon nanotubes produced in this way has generally been poorer than those made by arc-evaporation, but great improvements in the technique have been made in recent years. The big advantage of catalytic synthesis over arc-evaporation is that it can be scaled up for volume production. The third important method for making carbon nanotubes involves using a powerful laser to vaporise a metal-graphite target. This can be used to produce single-walled tubes with high yield [5].

There are lots of advantages of these productions methods, nevertheless we should mention the limitation of some experimental techniques. Methods for Bulk Synthesis of Carbon Nanotubes are in no way limited to supercapacitors, in which nanotubes provide a combination of high electrical conductivity and high surface area; field emitters, which require ordered arrays of nanotubes; and additives to plastics and rubbers that improve their electrical and thermal conductivity [3].

Isolated SWNT s (single-walled nanotubes) grown on quartz or silicon subtracts cannot be readily studied using high-resolution TEM. The sample must be dispersed, or grown, on a conducting surface to be examined. However, these limitations are not mentioned to downplay the importance of imaging methods.

Current use and application of nanotubes has mostly been limited to the use of bulk nanotubes, which is a mass of rather unorganized fragments of nanotubes. Bulk nanotube materials may never achieve a tensile strength similar to that of individual tubes, but such composites may nevertheless yield strengths sufficient for many applications. Bulk carbon nanotubes have already been used as composite fibers in polymers to improve the mechanical, thermal and electrical properties of the bulk product. Easton-Bell Sports, Inc. have been in partnership with Zyvex, using CNT technology in a number of their bicycle components - including flat and riser handlebars, cranks, forks, seatposts, stems and aero bars.

So, benefits of these techniques are that they are supported by big companies and technologies are well-known.

[1] Y. Zhu, C. Ke and H. D. Espinosa, Experimental Techniques for the Mechanical Characterization of One-Dimensional Nanostructures // Experimental Mechanics, Vol. 47, No. 1

[2] M.J.M. Daenen, R. de Fouw, B. Hamers, P.G.A. Janssen, K. Schouteden, M.A.J. Veld (ST), Wondrous World of Carbon Nanotubes, URL: http://students.chem.tue.nl/ifp03/default.htm

[3] Yury Gogotsi, Methods for Bulk Synthesis of Carbon Nanotubes, United States Patent Application 20080219913

[4] M. Meyyappan, Carbon nanotubes, 2004

[5] Peter Harris, Carbon nanotube science and technology, URL: http://www.personal.reading.ac.uk/~scsharip/tubes.htm